Question

Block 1, of mass m₁ = 0.650 kg , is connected over an ideal (massless and frictionless) pulley to block 2, of mass m₂, as shown. For an angle of θ = 30.0° and a coefficient of kinetic friction between block 2 and the plane of μ = 0.200, an acceleration of magnitude a = 0.450m/s² is observed for block 2.

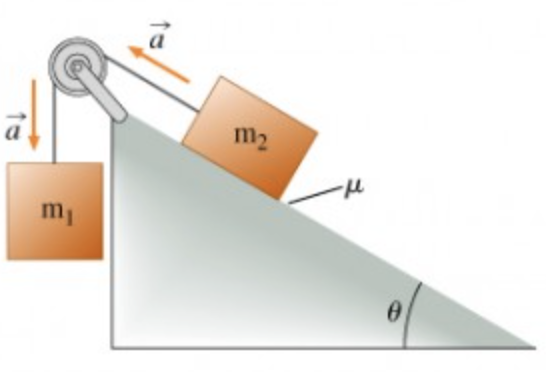
Part A

Find the mass of block 2, m₂.

Answer 1

Part A Answer

There are a couple of ways to solve this problem. One method is to have a look at the problem Two Masses a Pulley and an Inclined Plane. The problem linked gives you a formula to find the ratio of the masses m1 and m2. This formula is:

m1/m2 = (a + gsin(θ) + μgcos(θ)) / (g – a)

This gives us:

0.650/m2 = ((0.450 + 9.8 * sin(30)) + (0.200 * 9.8 * cos(30))) / (9.8 – 0.450)
0.650/m2 = (2.205 + 1.70) / (9.350)
0.650/m2 = 0.4176
0.650 = 0.4176m2
m2 = 1.56 kg

1.56 kg