Question

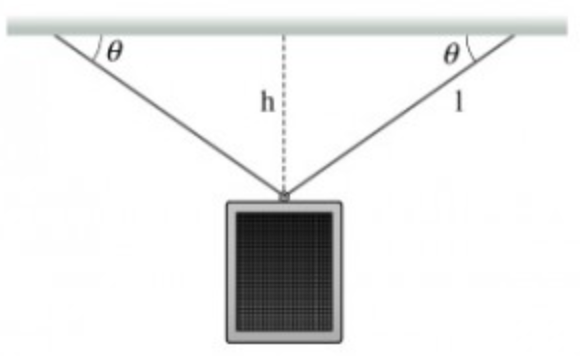
A loudspeaker of mass 24.0 kg is suspended a distance of h = 1.00 below the ceiling by two cables that make equal angles with the ceiling. Each cable has a length of l = 3.10.

Part A

What is the tension T in each of the cables?
Use 9.80 m/s² for the magnitude of the acceleration due to gravity.

Answer 1

Part A Answer

The tension will reflect forces in both the x and y-direction. The tension in the x-direction will have to cancel between the two cables, but the tension in the y-direction won’t. Overall, the tension will be greater than just the force due to gravity because it will reflect the x-contribution from each cable.

The force due to gravity is 9.8 * 24 = 235.2 N. This means each cable experiences 117.6 N of vertical tension (235.2 / 2). For the x-direction, we know that each cable's length is 3.10 m, and the height is 1.0 m. This means we can solve for the angle (sin(θ) = 1.0/3.10). So θ = 18.82°. The vertical component of the tension is Ty = Tsin(θ). Since we know that Ty = 235.2 N, we can solve for T. T is just 117.6 / sin(18.82°), or 365 N.

The answer is 365 N.