Question
In: Advanced Math
Find the values of sin θ, cos θ, and tan θ for the given right triangle. Give the exact values.
Find the values of sin θ, cos θ, and tan θ for the given right triangle. Give the exact values.
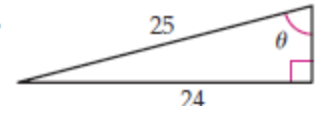
Solutions
Expert Solution
since,
\(\sin \theta=\frac{\text { height }}{\text { hypotenuse }}\)
Where height of the triangle is that side which is opposite to the angle \(\theta\)
Hence,
\(\sin \theta=\sqrt{\frac{24}{25}}\)
since,
\(\cos \theta=\frac{\text { base }}{\text { hypotenuse }}\)
Where base of the triangle is that side on which the angle is inclined
Hence,
$$ \begin{aligned} \cos \theta &=\frac{\sqrt{25^{2}-24^{2}}}{25} \\ &=\frac{\sqrt{625-576}}{25} \\ &=\frac{\sqrt{49}}{25} \\ &=\frac{7}{25} \end{aligned} $$
since,
\(\tan \theta=\frac{\text { height }}{\text { base }}\)
Where height of the triangle is that side which is opposite to the angle \(\theta\) and base of the triangle
is that side on which the angle is inclined
Hence,
\(\tan \theta=\sqrt{\frac{24}{7}}\)
Related Solutions
Suppose θ is an acute angle in a right triangle. Given tan(θ)=1/5, evaluate: 1-sin^2(θ)
3. Let g(θ) = 2 cos(θ) + sin(2θ) . Find the absolute maximum and minimum values...
Express sin 6θ as a polynomial in sin θ and cos θ.
Given H = (3R^2/ sin θ) aθ + 54 R cos θ aφ A/m (a) Find...
Find the values of the trigonometric functions of θ from the information given. cos(θ) = −3/11,...
1) Find the critical numbers of the function. f(θ) = 16 cos θ + 8 sin^2 θ
If 3/(2+ cos θ+i sin θ) = a+ib, then [(a-2)2+b2] is
Simplify. Do not give trig values. 1) 2 sin40 cos 40 2) 10 sin 18 cos...
a.)Find the length of the spiral r=θ for 0 ≤ θ ≤ 2 b.)Find the exact...
Find two values of θ that satisfy the given equation, where θ∈[0,2π], is measured in radians....
- QUESTION 15 We need to write a function that calculates the power of x to n...
- Your neighbor tells you that her nine-year-old son has been having behavior and academic problems in...
- I can’t get my code to work and/or finish it. Please fix. Below are code instructions...
- Three entrepreneurs plan to open a copy shop. It costs $5000 per year to rent a...
- I understand how electrons initially move into another's vicinity, but nowhere can I find a fathomable...
- The work climate has proven to be an effective measuring stick to successful performance. Tell what...
- 1b. Explain each of the following with an example in two languages of your choice for...
 Dr. OWL answered 4 years ago
Dr. OWL answered 4 years ago