Question

For each phase change, determine the sign of \({\Delta H}\) and \(\Delta S\) Sublimation, Freezing,Condensation, Deposition, Boiling, Melting

a) \(+\Delta \mathrm{H},+\Delta \mathrm{S}\)

b) \(-\Delta \mathrm{H},-\Delta \mathrm{S}\)

c) \(+\Delta H,-\Delta S\)

d) \(-\Delta H,+\Delta S\)

Put the phase change to the correctsign of \(\Delta \mathrm{H}\) and \(\Delta \mathrm{S}\)

Answer 1

Concepts and reason

The change in energy \((\Delta H)\) during phase change or a reaction is known as Enthalpy. When energy is absorbed during reaction or phase change, the sign for enthalpy is taken as positive. When energy is released during reaction or phase change, the sign for enthalpy is taken as negative. The change in entropy (degree of randomness, \(\Delta S\) ) during phase change or a reaction is known as Entropy. When the degree of randomness is increased, it is given a positive sign, and when the degree of randomness is decreased, it is given a negative sign. In the given question, you need to determine the signs of \(\Delta H\) and \(\Delta S\) for various processes mentioned in the question.

Fundamentals

Sublimation - The process in which a solid-state substance is directly converted into the gaseous state is termed sublimation. Freezing - The process in which a substance in the liquid state is converted into the solid-state is termed freezing. Condensation - The process in which a substance in the gaseous state is converted into a liquid state is termed condensation. Deposition - The process in which a substance in the gaseous state is directly converted into the solid-state is termed deposition. Boiling - The process in which a substance in the liquid state is converted into the gaseous state is termed boiling. Melting - The process in which a solid-state substance is converted into a liquid state is termed melting.

 

For sublimation, the change in energy is positive, and the change in randomness is also positive.

The sign of \(\Delta H\) and \(\Delta S\) for the sublimation process is

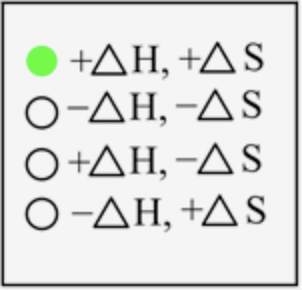
In sublimation, the solid-state is converted directly into a gaseous state, which means energy is required to break the interaction between the solid-state to change it into a gaseous state. Thus, energy is absorbed here, and the sign is positive. The degree of randomness increases on moving from solid to a gaseous state; therefore, entropy is also positive.

 

For freezing, the change in energy is negative, and the change in randomness is also negative.

The sign of \(\Delta H\) and \(\Delta S\) for the freezing process is

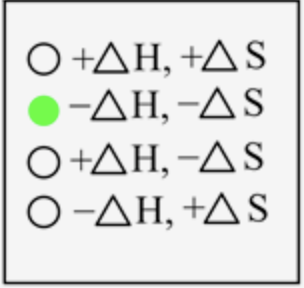
In freezing, the liquid state is converted into a solid-state, which means energy is released when atoms come closer to each other. Thus, energy is released here, and the sign is negative. The degree of randomness decreases when moving from liquid to solid-state; therefore, entropy is also negative.

 

For condensation, the change in energy is negative, and the change in randomness is also negative.

The sign of \(\Delta H\) and \(\Delta S\) for the condensation process is

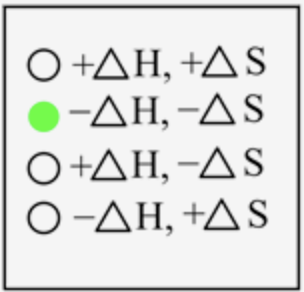
In condensation, the gaseous state is converted into a liquid state, which means energy is released when atoms come closer to each other. Thus, energy is released here, and the sign is negative. The degree of randomness decreases when moving from gas to liquid state; therefore, entropy is also negative.

 

For deposition, the change in energy is negative, and the change in randomness is also negative.

The sign of \(\Delta H\) and \(\Delta S\) for the deposition process is

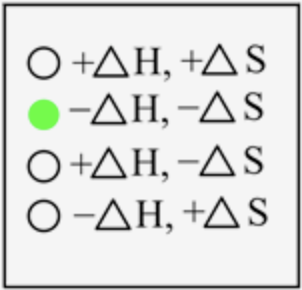
In a deposition, the gaseous state is directly converted into a solid-state, which means energy is released when atoms come closer to each other. Thus, energy is released here, and the sign is negative. The degree of randomness decreases when moving from gas to solid-state; therefore, entropy is also negative.

 

For boiling, the change in energy is positive, and the change in randomness is also positive.

The sign of \(\Delta H\) and \(\Delta S\) for the boiling process is

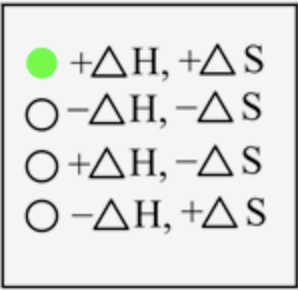
In boiling, the liquid state is converted into a gaseous state, which means energy is required to break the interaction between the liquid state to change it into a gaseous state. Thus, energy is absorbed here, and the sign is positive. The degree of randomness increases on moving from liquid to gaseous state; therefore, entropy is also positive.

 

For melting, the change in energy is positive, and the change in randomness is also positive.

The sign of \(\Delta H\) and \(\Delta S\) for the melting process is

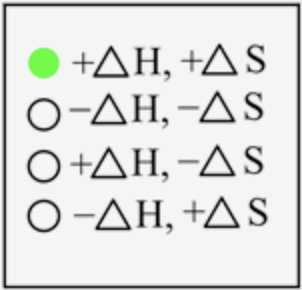
In melting, the solid-state is converted into a liquid state, which means energy is required to break the interaction between the solid-state to change it into a liquid state. Thus, energy is absorbed here, and the sign is positive. The degree of randomness increases on moving from solid to liquid state; therefore, entropy is also positive.