Question

9)

According to a census that was conducted five years ago, the mean annual household salary in the city of Townsburg was $40,084. The mayor of Townsburg believes that there has been an economic upturn in the city since then. He appoints a team to test for evidence that the mean annual household salary in Townsburg has increased. The teams surveys a sample of 41 households. The sample has a mean annual salary of $40,862 with a standard deviation of $2688.   

Test for evidence that the mean annual household salary in Townsburg has increased during the last five years. Use a level of significance of α = 0.05.

p = 0.0356
We do not reject the null hypothesis. There is not sufficient evidence at the 5% level of significance to suggest that the mean annual household salary in Townsburg has increased in the last five years.

p = 0.0712
We do not reject the null hypothesis. There is not sufficient evidence at the 5% level of significance to suggest that the mean annual household salary in Townsburg has increased in the last five years.

p = 0.0712
We reject the null hypothesis. There is sufficient evidence at the 5% level of significance to suggest that the mean annual household salary in Townsburg has increased in the last five years.

p = 0.0356
We reject the null hypothesis. There is sufficient evidence at the 5% level of significance to suggest that the mean annual household salary in Townsburg has increased in the last five years.

p = 0.0319
We reject the null hypothesis. There is sufficient evidence at the 5% level of significance to suggest that the mean annual household salary in Townsburg has increased in the last five years.

p = 0.0319
We do not reject the null hypothesis. There is not sufficient evidence at the 5% level of significance to suggest that the mean annual household salary in Townsburg has increased in the last five years.

10)

A company produces ball bearings which have a mean diameter of 7.33 mm. After replacing some parts in their production line, the lead engineer wants to check to see if there has been a change in the diameters of the ball bearings being produced. A sample of 38 ball bearings is checked, resulting in a sample mean diameter of 7.27 mm with a sample standard deviation of 0.17 mm.

Test for evidence that there has been a change (in either direction) in the mean diameter of the ball bearings. Use a level of significance of α = 0.05.

p = 0.0180
We reject the null hypothesis. There is sufficient evidence at the 5% level of significance to suggest that their has been a change in the mean diameter of the ball bearings.

p = 0.0148
We reject the null hypothesis. There is sufficient evidence at the 5% level of significance to suggest that their has been a change in the mean diameter of the ball bearings.

p = 0.0360
We do not reject the null hypothesis. There is not sufficient evidence at the 5% level of significance to suggest that their has been a change in the mean diameter of the ball bearings.

p = 0.0148
We do not reject the null hypothesis. There is not sufficient evidence at the 5% level of significance to suggest that their has been a change in the mean diameter of the ball bearings.

p = 0.0360
We reject the null hypothesis. There is sufficient evidence at the 5% level of significance to suggest that their has been a change in the mean diameter of the ball bearings.

p = 0.0180
We do not reject the null hypothesis. There is not sufficient evidence at the 5% level of significance to suggest that their has been a change in the mean diameter of the ball bearings.

Answer 1

9.

One sample t test using Minitab:

This is Right tailed test :

Click Stat > Basic Statistics > 1-Sample t... on the top menu, as shown below:

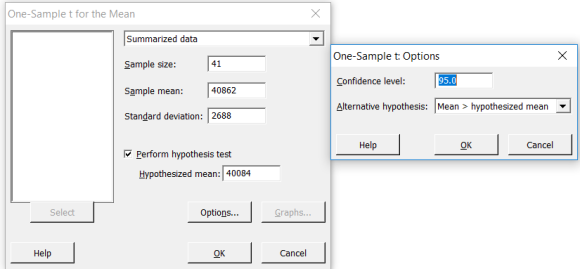
You will be presented with the following 1-Sample t (Test and Confidence Interval) dialogue box:

Click the OK button. The output that Minitab produces is shown below.

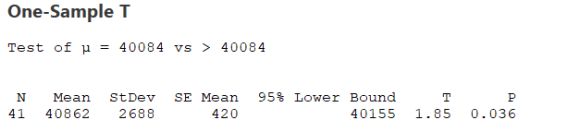
Correct Option is

p = 0.0356
We do not reject the null hypothesis. There is not sufficient evidence at the 5% level of significance to suggest that the mean annual household salary in Townsburg has increased in the last five years.

Post remaining questions sapeartely. Hope this will be helpful. Thanks and God Bless You :)