Question
In: Statistics and Probability
Heights are generally normally distributed. Men have a mean of 69.5 inches and standard deviation 2.4...
Heights are generally normally distributed. Men have a mean of 69.5 inches and standard deviation 2.4 inches. Women have a mean of 63.8 inches and standard deviation 2.6 inches. The US Air Force has a height requirement for their pilots to be between 64 inches and 77 inches.
Make sure you are rounding z-scores properly to two places.
Part A: Find the two z-scores for women who meet this height requirement z = (smaller value) and z = (larger value)
Part B: Using the z-scores from part A, find the proportion of women who meet the height requirement. Give this answer as a decimal.
Part C: Find the two z-scores for men who meet this height requirement z = (smaller value) and z = (larger value)
Part D: Using the z-scores from part C, find the proportion of men who meet the height requirement. Give this answer as a decimal.
Part E: If the height requirement were changed to exclude only the shortest 1% of women and tallest 1% of men, what are the new heights? The short value would be in inches and the tall value would be in inches. Please give these two values rounded properly to one decimal place.
Solutions
Expert Solution
The US Air Force has a height requirement for its pilots to be between 64 inches and 77 inches.
A) women height is normally distributed with 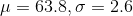
the two z-scores for women who meet this height requirement
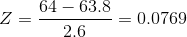
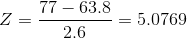
B) The proportion of women who meet the height requirement =
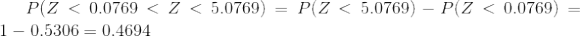
C) Men have a mean of 69.5 inches and a standard deviation of 2.4 inches.
the two z-scores for men who meet this height requirement
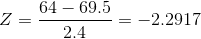
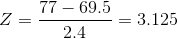
D) the proportion of men who meet the height requirement. =

E) Let A be the value below which the proportion of women is 0.01
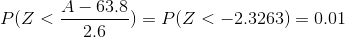
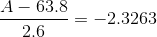
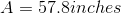
Let B be the value above which the proportion of men is 0.01

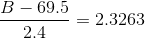
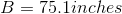
Related Solutions
Men’s heights are normally distributed with a mean of 69.5 inches and a standard deviation of...
The heights of adult men in America are normally distributed, with a mean of 69.5 inches...
The heights of adult men in America are normally distributed, with a mean of 69.5 inches...
Assume heights of men are normally distributed with a mean of 69.3 inches with a standard...
Assume heights of men are normally distributed with a mean of 69.3 inches with a standard...
The heights of 18-year-old men are approximately normally distributed with mean 68 inches and standard deviation...
The heights of 18-year-old men are approximately normally distributed with mean 68 inches and standard deviation...
Heights of fences are normally distributed with a mean of 52 inches and a standard deviation...
Heights of women are normally distributed with a mean of 63.8 inches and a standard deviation...
Men’s heights are normally distributed with a mean of 72 inches and a standard deviation of...
- British Columbia Lumber has a raw lumber division and a finished lumber division. The variable costs...
- Discussion 1 – due 23 February 2020, 11:55 PM ECT (5% of coursework marks) Provide Sue...
- Describe how at least one of the laws of thermodynamics relates to your room, or the...
- Instruction: The table consists of information about 2 competing investments. ...
- This question is about concentration measurements and its effect on E and K. Cu/Cu^+2 // Ag/Ag^+2...
- Agree/Disagree and Why? Integer linear programs involve a class of problems that are modeled as linear...
- This assignment will be submitted to Turnitin®. Instructions Instruction: Complete your essay in a new MS...
 orchestra answered 2 years ago
orchestra answered 2 years ago