Question
In: Mechanical Engineering
The jet transport B is flying north with a velocity
The jet transport \(B\) is flying north with a velocity \(v_{\mathrm{B}}=600 \mathrm{~km} / \mathrm{h}\) when a smaller aircraft \(A\) passes underneath the transport headed in the \(60^{\circ}\) direction shown. To passengers in \(B\), however, \(A\) appears to be flying sideways and moving east. Determine the actual velocity of \(A\) and the velocity which \(A\) appears to have relative to \(B\).
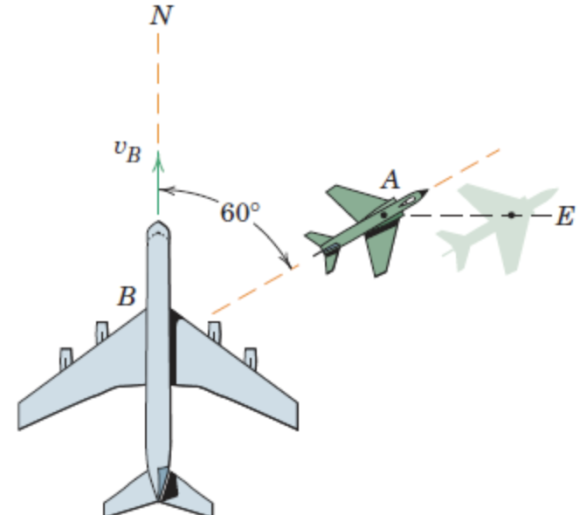
Solutions
Expert Solution
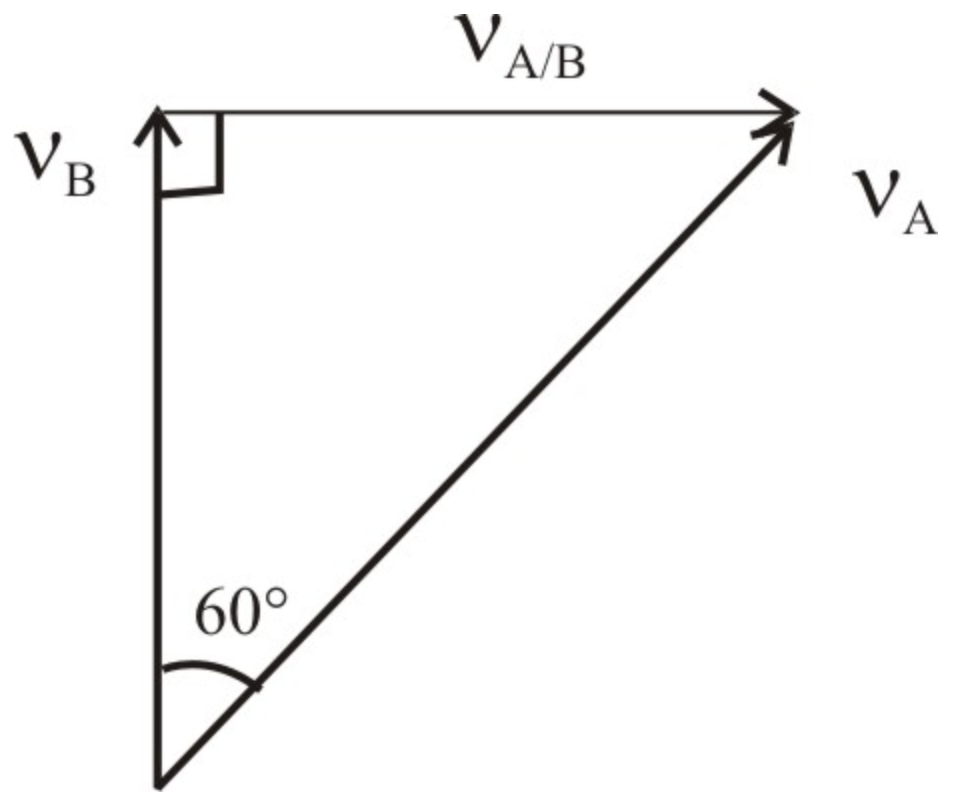
Given:
\(v_{B}=600 \mathrm{~km} / \mathrm{h}\)
From the vector triangle,
\(v_{A}=\frac{v_{B}}{\cos 60^{\circ}}\)
\(=\frac{600}{\cos 60}\)
\(=1200\)
\(\therefore v_{A}=1200 \mathrm{~km} / \mathrm{h}\)
The velocity which A appears relative to B,
\(v_{\frac{A}{B}}=v_{B} \tan 60^{\circ}\)
\(=600 \times \tan 60^{\circ}\)
\(=1039.23\)
\(\therefore v_{\frac{A}{B}}=1039.23 \mathrm{~km} / \mathrm{h}\)
\( v_{A}=1200 \mathrm{~km} / \mathrm{h}\)
\( v_{\frac{A}{B}}=1039.23 \mathrm{~km} / \mathrm{h}\)
Related Solutions
An airplane is flying with a velocity of v0 at an angle of α above the...
An airplane is flying with a velocity of v0 at an angle of α above the...
A plane flying at 600 km / h to the north is held by a wind...
(1) Is a CEO using a corporate jet (instead of flying commercial) a sign of severe...
A jet is flying with an air speed of 500 m/s. A parcel is dropped out...
An eagle is flying horizontally at the altitude of 400 ft with a velocity of 16...
An airplane is flying with a velocity of 240 m/s at an angle of 30.0° with...
A 21500 kg jet airplane is flying through some high winds. At some point in time,...
1. A commercial airliner is flying at 450 mph, 20° east of north, relative to the...
An airplane flying in the air with a constant velocity of 240 m/s horizontally experiences a...
- A spherical object has an outside diameter of 60.0cm . Its outer shell is composed of...
- Write the code for binary min heap in c++ .
- Starting with a 30% (w/w) solution of hydrogen peroxide, how many mL would you need to...
- An object with a density of 761.0 kg/m3 and a mass of 1399.0 kg is thrown...
- British Columbia Lumber has a raw lumber division and a finished lumber division. The variable costs...
- Discussion 1 – due 23 February 2020, 11:55 PM ECT (5% of coursework marks) Provide Sue...
- Describe how at least one of the laws of thermodynamics relates to your room, or the...
 Dr. OWL answered 4 years ago
Dr. OWL answered 4 years ago