Question
In: Physics
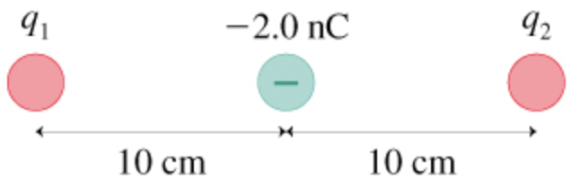
In (Figure 1), charge q2 experiences no net electric force. What is q1?
In (Figure 1), charge q2 experiences no net electric force. What is q1?
Solutions
Expert Solution
Concepts and reason
The concept required to solve this problem is electric force and Coulomb's law. Initially, obtain the expression for net electric force on \(q_{2}\) by using expression for electric force and knowing direction of force due to charge \(q_{1}\) and \(-2.0 \mathrm{n} \mathrm{C}\) then find the charge \(q_{1}\).
Fundamentals
According to Coulomb's law, the electric force between two charged particles is directly proportional to the product of their magnitude of charges and inversely proportional to the square of the distance between them. In equation form, Coulomb's law can be stated as \(F=\frac{k q_{1} q_{2}}{r^{2}}\)
Here, \(q_{1}\) and \(q_{2}\) are the charges separated by a distance \(r\) and \(k\) is the Coulomb's constant.
Use Coulomb’s law, to solve for the magnitude of the force of one charge on another. Also use the idea that charges of the same signs repel while charges of opposite signs attract.
Distance between charges \(q_{1}\) and \(q_{3}\) is,
$$ \begin{array}{c} r_{1}=10 \mathrm{~cm} \\ =10 \times 10^{-2} \mathrm{~m} \end{array} $$
Distance between charges \(q_{3}\) and \(q_{2}\) is,
$$ \begin{array}{c} r_{2}=10 \mathrm{~cm} \\ =10 \times 10^{-2} \mathrm{~m} \end{array} $$
So distance between charges \(q_{1}\) and \(q_{2}\) is,
\(r=r_{1}+r_{2}\)
Substitute \(10 \times 10^{-2} \mathrm{~m}\) for \(r_{1}\) and \(r_{2}\) in equation \(r=r_{1}+r_{2}\) as follows:
$$ \begin{array}{c} r=10 \times 10^{-2} \mathrm{~m}+10 \times 10^{-2} \mathrm{~m} \\ =20 \times 10^{-2} \mathrm{~m} \\ =0.2 \mathrm{~m} \end{array} $$
The net force on \(q_{2}\) due to \(q_{1}\) and \(q_{3}\) is given by, \(F_{\text {neton } q 2}=F_{q 1 \text { on } q 2}+F_{q 3 \text { on } q 2}\)
Here, \(F_{q 3 \text { on } q 2}\) is the force due to \(q_{3}\) on \(q_{2}\) and \(F_{q 1 \text { on } q 2}\) is the force due to \(q_{1}\) on \(q_{2}\) The force on \(q_{2}\) due to \(q_{1}\) is \(F_{q 1 \mathrm{on} q 2}=\frac{k q_{1} g_{2}}{(r)^{2}}\)
Substitute \(0.2 \mathrm{~m}\) for \(r\) in equation \(F_{q 1 \mathrm{on} q 2}=\frac{k q_{1} q_{2}}{(r)^{2}}\) as follows:
\(F_{q 1 \mathrm{on} q 2}=\frac{k q_{1} q_{2}}{(0.2 \mathrm{~m})^{2}}\)
The force on \(q_{2}\) due to \(q_{3}\) is \(F_{q 3 \text { on } q 2}=\frac{k q_{3} q_{2}}{\left(r_{2}\right)^{2}}\)
Substitute \(0.1 \mathrm{~m}\) for \(r_{2}\) and \(-2 \times 10^{-9} C\) for \(q_{3}\) in equation \(F_{q 3 \mathrm{on} q 2}=\frac{k q_{3} q_{2}}{\left(r_{2}\right)^{2}}\) as follows:
\(F_{q 3 \mathrm{on} q 2}=\frac{k q_{2}\left(-2 \times 10^{-9} C\right)}{(0.2 \mathrm{~m})^{2}}\)
Substitute \(\frac{k q_{2}\left(-2 \times 10^{-9} C\right)}{(0.2 \mathrm{~m})^{2}}\) for \(F_{q 3 \mathrm{on} q 2}\) and \(\frac{k q_{1} q_{2}}{(0.2 \mathrm{~m})^{2}}\) for \(F_{q_{1} \text { on } q 2}\) in equation \(F_{\text {neton } q 2}=F_{q_{1} \text { on } q_{2}}+F_{q 3 \text { on } q 2}\) as follows:
$$ \begin{array}{l} F_{\text {neton } q_{2}}=\frac{k q_{1} q 2}{(0.2 \mathrm{~m})^{2}}+\frac{k\left(-2 \times 10^{-9} \mathrm{C}\right) q_{2}}{(0.10 \mathrm{~m})^{2}} \\ \quad=\frac{k q_{1} q_{2}}{(0.2 \mathrm{~m})^{2}}-\frac{k\left(2 \times 10^{-9} \mathrm{C}\right) q_{2}}{(0.10 \mathrm{~m})^{2}} \end{array} $$
Here assume that the charge \(q_{2}\) is positive. Considering the forces on positive charge \(q_{2}\). The other positive charge,
\(q_{1}\), exerts a repulsive force \(q_{2}\) that pushes \(q_{2}\) away from \(q_{1}\), that is, to the right. The negative charge \(q_{3}\) exerts an
attractive force on \(q_{2}\) that pulls \(q_{2}\) toward \(q_{3}\), that is, to the left. For the net force on \(q_{2}\) to be zero, these two forces must have the same magnitude.
Equate the net force on \(q_{2}\) to zero, \(F_{\text {neton } q_{2}}=0\) \(k q_{2}\left[\frac{q 1}{(0.2 \mathrm{~m})^{2}}-\frac{2 \times 10^{-9} \mathrm{C}}{(0.10 \mathrm{~m})^{2}}\right]=0\)
Rearrange the above equation as follows:
$$ \begin{array}{c} {\left[\frac{q_{1}}{(0.2 \mathrm{~m})^{2}}-\frac{2 \times 10^{-9} \mathrm{C}}{(0.10 \mathrm{~m})^{2}}\right]=0} \\ \frac{q_{1}}{(0.2 \mathrm{~m})^{2}}=\frac{2 \times 10^{-9} \mathrm{C}}{(0.10 \mathrm{~m})^{2}} \\ q_{1}=\frac{(0.2 \mathrm{~m})^{2} \times\left(2 \times 10^{-9} \mathrm{C}\right)}{(0.10 \mathrm{~m})^{2}} \\ =8 \times 10^{-9} \mathrm{C} \end{array} $$
Convert the units of charge from Coulomb's to Nano Coulombs as follows:
$$ \begin{array}{c} q_{1}=8 \times 10^{-9} \mathrm{C}\left(\frac{1 \mathrm{nC}}{10^{-9} \mathrm{C}}\right) \\ =8 \mathrm{nC} \end{array} $$
Charge \(q_{1}\) is \(8 \mathrm{nC}\).
The Charge \(q_{1}\) is \(8 \mathrm{nC}\).
The charged particles are point charges. The charge \(q_{2}\) is in static equilibrium, so the net force on \(q_{2}\) is zero.
The Charge \(q_{1}\) is \(8 \mathrm{nC}\).
Related Solutions
What is the magnitude of the electric force on charge A in the figure?
A 30 nC charge experiences a 0.035 N electric force.
1)What is The charge on 6 alpha particles? 2) Suppose the charge q2 in the figure...
Find the direction and magnitude of the net electrostatic force exerted on the point charge q2...
What is the magnitude of the net force on the first wire in (figure 1)?
An electron is released in a uniform electric field, and it experiences an electric force of...
A charge of q1 = 4.0µC is at the origin and a charge q2 = 2.0µC...
In the figure particle 1 of charge q1 = 0.93 μC and particle 2 of charge...
A charge Q1 = +9μC is located at the origin and a second charge Q2 =...
Particle 1 of charge q1 = 0.96 μC and particle 2 of charge q2 = -3.02...
- An object with a density of 761.0 kg/m3 and a mass of 1399.0 kg is thrown...
- British Columbia Lumber has a raw lumber division and a finished lumber division. The variable costs...
- Discussion 1 – due 23 February 2020, 11:55 PM ECT (5% of coursework marks) Provide Sue...
- Describe how at least one of the laws of thermodynamics relates to your room, or the...
- Instruction: The table consists of information about 2 competing investments. ...
- This question is about concentration measurements and its effect on E and K. Cu/Cu^+2 // Ag/Ag^+2...
- Agree/Disagree and Why? Integer linear programs involve a class of problems that are modeled as linear...
 Dr. OWL answered 4 years ago
Dr. OWL answered 4 years ago