Question

In the article “Explaining an Unusual Allergy,” appearing on the Everyday Health Network, Dr. A Feldweg explained that allergy to sulfites is usually seen in patients with asthma. The typical reaction is a sudden increase in asthma symptoms after eating a food containing sulfites. Studies are performed to estimate the percentage of the nation’s 10 million asthmatics who are allergic to sulfites. In one survey, 38 of 500 randomly selected U.S. asthmatics were found to be allergic to sulfites.
(Source: Elementary Statistics, Weiss, 8th Edition)

If you were to construct a 98% confidence interval for the proportion of all U.S. asthmatics who are allergic to sulfites, what is the margin of error used to calculate the interval? (use the standard error found in problem 1, round your critical value to the hundredths place, and round the margin of error to the thousandths place)

Answer 1

Solution :

Given that,

n = 500

x = 38

Point estimate = sample proportion = 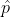= x / n = 38 / 500 =0.076

1 - 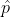= 1 - 0.076 =0.924

At 98% confidence level

= 1 - 98%

=1 - 0.98 =0.02

/2 = 0.01

Z/2 = Z_{0.01 = 2.33}

Margin of error = E = Z _{/ 2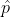} * (( * (1 - 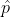)) / n)

= 2.33 (((0.076 * 0.924) / 500)

= 0.028

A 98% confidence interval for population proportion p is ,

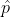- E < p < 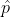+ E

0.076 - 0.028< p < 0.076 +0.028

0.048< p < 0.104

The 98% confidence interval for the population proportion p is :(0.048 , 0.104)