Question

The weekly demand for a certain drink, in thousands of liters, from a local chain of efficiency stores, is a continuous random variable X with cumulative distribution function ?(?) = (? − 1)2 , 1 < ? < 2.

a) Find P( 1.25 < X < 1.75).

b) Find the probability density function of X.

Answer 1

The cumulative distribution function of the random variable X is given by,

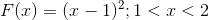
(a) Find P(1.25<X<1.75)

Solution::

We know that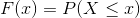

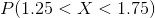
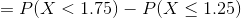
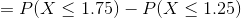[ Since X is a continuous random variable]

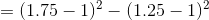
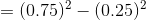
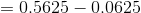
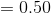
The probability is 0.50

(b) Find the probability density function of X.

Solution::

To find the probability density function of X, we need to derivatives F(x) with respect to x.

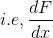
Here,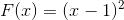

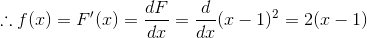
The probability density function of the random variable X is given by,