Question
In: Economics
Consider the regression we know that f there is a strong linear correlation between X and...
Consider the regression we know that f there is a strong linear correlation between X and Z, then it is more likely that the t-test statistics get smaller. Show and explain how. Explain what happens if t-statistics get smaller.
Solutions
Expert Solution
1. If there is a strong correlation between X and Z, then
Cov(x,z)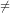 0
0
The t statistic is given by :
t =  , where
, where  is the hypothesised value
is the hypothesised value
and ,
s.e(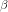 )
=
)
=  and variance is
and variance is
var(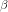 )
=
)
=
where Rk2 is the coefficient of determination of auxiliary regression of xk on other regressors.
- When X and Z are uncorrelated, Rk2=0 and
var(
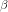 )=
)=
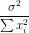
- When X and Z are correlated, then
Rk2
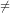 0
0
Then , since R2 is less than or equal to 1, the denominator decreases (since now it is multiplied by a number less than 1) and hence the variance and consequently the standard error of coefficient increases.
Due to increase in standard error, the denominator in t statistic, i.e
t =  will increase and hence the t statistics becomes
smaller.
will increase and hence the t statistics becomes
smaller.
Hence, due to strong linear correlation between X and Z, the standard error increases and hence the denominator of t statistics increase result in a smaller t statistic.
2. When the t statistics gets smaller, our standard errors increase while the confidence intervals become wider.
Let,
H0: 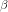 =0
=0
H1: 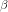
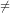 0
0
We reject the null hypothesis based on t test when: t > tcritical
Due to presence of correlation between regressors, t statistics become smaller and hence probability of rejecting the null hypothesis will reduce ( also due to increase in confidence intervals which are acceptance regions). It is likely that we will accept the null hypothesis and the coefficient will come out to be statistically insignificant.
This is the problem of multicollinearity caused by correlation between regressors which results in few significant t ratios.
Related Solutions
The Pearson correlation coefficient (r) equals one when there is strong linear relationship between x and...
Regression and correlation analysis both describe the strength of linear relationships between variables. Consider the concepts...
Consider the function f(x)=2e2sin(x). In this question, we will first use a linear approximation to estimate...
We know that a linear regression model is limited in its power to project into the...
Consider the following problem involving linear correlation and linear regression: How much should a healthy calf...
So, as we look at Linear Regression and correlation this week, please find provide an example...
1. True or False: If we know that f(x) is increasing on an interval, then f...
You are developing a simple linear regression analysis model. The simple correlation coefficient between y and...
QUESTION BLOCK: Linear Regression and R-squared If we know the value of b, the slope of...
QUESTION BLOCK: Linear Regression and R-squared If we know the value of b, the slope of...
- Write the code for binary min heap in c++ .
- Starting with a 30% (w/w) solution of hydrogen peroxide, how many mL would you need to...
- An object with a density of 761.0 kg/m3 and a mass of 1399.0 kg is thrown...
- British Columbia Lumber has a raw lumber division and a finished lumber division. The variable costs...
- Discussion 1 – due 23 February 2020, 11:55 PM ECT (5% of coursework marks) Provide Sue...
- Describe how at least one of the laws of thermodynamics relates to your room, or the...
- Instruction: The table consists of information about 2 competing investments. ...
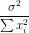
 Rahul Sunny answered 1 year ago
Rahul Sunny answered 1 year ago