Question
In: Physics
) A 1.53-kg bucket hangs on a rope wrapped around a pulley of mass 7.07 kg...
) A 1.53-kg bucket hangs on a rope wrapped around a pulley of mass 7.07 kg and radius 66 cm. This pulley is frictionless in its axle, and has the shape of a solid uniform disk. After the bucket has been released, what is the angular acceleration of the pulley?
Solutions
Expert Solution
Gravitational acceleration = g = 9.81 m/s2
Mass of the bucket = m = 1.53 kg
Mass of the pulley = M = 7.07 kg
Radius of the pulley = R = 66 cm = 0.66 m
Moment of inertia of the pulley = I
I = MR2/2
I = (7.07)(0.66)2/2
I = 1.54 kg.m2
Tension in the rope = T
Angular acceleration of the pulley = 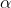
Acceleration of the bucket = a
a = 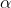 R
R
For the bucket,
ma = mg - T
T = mg - ma
T = m(g - 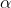 R)
R)
For the pulley,
I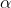 = TR
= TR
I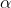 = m(g -
= m(g -
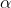 R)R
R)R
(1.54)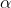 =
(1.53)(0.66)(9.81 - 0.66
=
(1.53)(0.66)(9.81 - 0.66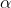 )
)
1.54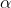 = 9.906 -
0.666
= 9.906 -
0.666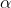
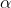 = 4.489
rad/s2
= 4.489
rad/s2
Angular acceleration of the pulley = 4.489 rad/s2
Related Solutions
A bucket of water of mass 16.0 kg is suspended by a rope wrapped around a...
A rope of negligible mass hangs vertically on each side of a pulley. The pulley, which...
A rope of negligible mass is wrapped around a 225-kg solid cylinder of radius 0.400 m....
A lightweight rope is wrapped around a 100-lb drum, passes over a frictionless pulley, and is...
A rope is wrapped around the rim of a large uniform solid disk of mass 155...
A 3.2 kg box hangs on a rope without mass and that does not stretch, the...
a bucket of water with total mass 20 kg is attached to. a rope, which in...
A string is wrapped around a pulley with a radius of 2.0 cm and no appreciable...
A 61.0 kg box hangs from a rope. What is the tension in the rope if:...
A 55.0 kg box hangs from a rope. What is the tension in the rope if:...
- Write the code for binary min heap in c++ .
- Starting with a 30% (w/w) solution of hydrogen peroxide, how many mL would you need to...
- An object with a density of 761.0 kg/m3 and a mass of 1399.0 kg is thrown...
- British Columbia Lumber has a raw lumber division and a finished lumber division. The variable costs...
- Discussion 1 – due 23 February 2020, 11:55 PM ECT (5% of coursework marks) Provide Sue...
- Describe how at least one of the laws of thermodynamics relates to your room, or the...
- Instruction: The table consists of information about 2 competing investments. ...
 genius_generous answered 2 years ago
genius_generous answered 2 years ago