Question

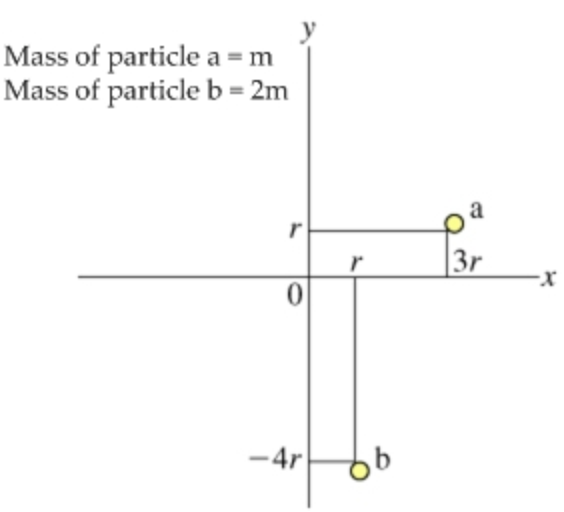
Find the moment of inertia Ix of particle a with respect to the x-axis (that is, if the x-axis is the axis of rotation), the moment of inertia Iy of particle a with respect to the y axis, and the moment of inertia Iz of particle a with respect to the z-axis (the axis that passes through the origin perpendicular to both the x and y axes).

Express your answers in terms of m and r separated by commas.

Answer 1

Concepts and reason

The concept required to solve the problem is the moment of inertia. Use the mass and the distance from \(\mathrm{x}\) axis to calculate the moment of inertia with respect to \(\mathrm{x}\) axis. Use the mass and the distance from y axis to calculate the moment of inertia with respect to y axis. Calculate the sum of the moment of inertias with respect to \(\mathrm{x}\) and \(\mathrm{y}\) axis to calculate the moment of inertia with respect to \(\mathrm{z}\) axis.

Fundamentals

The moment of inertia of the object is, \(I=M R^{2}\)

Here, \(M\) is the mass of the object and \(R\) is the perpendicular distance.

 

(C. 1) The moment of inertia of particle is, \(I=M R^{2}\)

The mass of particle a is \(m\) and the perpendicular distance of particle a from \(\mathrm{x}\) axis is \(r\). Substitute \(m\) for \(M\) and \(r\) for \(R\). The moment of inertia of particle a with respect to \(\mathrm{x}\) axis is, \(I_{x}=m r^{2}\)

Part C.1 The moment of inertia of particle a with respect to \(\mathrm{x}\) axis, \(I_{x},\) is \(\mathrm{mr}^{2}\)

The moment of inertia of the particle a with respect to x axis is the product of mass of particle a and the square of perpendicular distance from \(\mathrm{x}\) axis.

 

(C. 2) The moment of inertia of particle is, \(I=M R^{2}\)

The mass of particle a is \(m\) and the perpendicular distance of particle a from y axis is \(3 r\). Substitute \(m\) for \(M\) and \(3 r\) for \(R\). The moment of inertia of particle a with respect to y axis is,

$$ I_{y}=m(3 r)^{2} $$

\(=9 m r^{2}\)

Part C.2 The moment of inertia of particle a with respect to \(\mathrm{y}\) axis, \(I_{y},\) is \(9 \mathrm{mr}^{2}\)

The moment of inertia of the particle a with respect to \(\mathrm{y}\) axis is directly proportional to mass of particle \(a\) and the square of perpendicular distance from y axis.

 

(C.3) The moment of inertia of particle a with respect to \(z\) axis is, \(I_{z}=I_{x}+I_{y}\)

Substitute \(m r^{2}\) for \(I_{x}\) and \(9 m r^{2}\) for \(I_{y}\). The moment of inertia of the particle with respect to \(z\) axis is,

\(I_{z}=m r^{2}+9 m r^{2}\)

$$ =10 m r^{2} $$

Part C.3 The moment of inertia of particle a with respect to \(z\) axis, \(I_{z},\) is \(10 m r^{2}\)

The moment of inertia of the particle a with respect to \(z\) axis is the sum of the moment of inertia of particle a with respect to x axis and the sum of the moment of inertia of particle a with respect to y axis.

 

Part C.1 The moment of inertia of particle a with respect to \(\mathrm{x}\) axis, \(I_{x},\) is \(\mathrm{mr}^{2}\)

Part \(\mathrm{C} .2\) The moment of inertia of particle a with respect to \(\mathrm{y}\) axis, \(I_{y},\) is \(9 \mathrm{mr}^{2}\)

Part \(C .3\) The moment of inertia of particle a with respect to \(z\) axis, \(I_{z},\) is \(10 m r^{2}\)