Question

1. Consider a simplified one-dimensional laminar flame, such as that discussed in the classroom. The earliest description of a laminar flame is that of Mallard and Le Chatelier in 1883. Assume that:

a. 1-D, constant area, steady flow,

b. kinetic and potential energies, viscous shear work, and thermal

radiation are neglected,

c. pressure is constant,

d. diffusion of heat and mass are governed by Fourier’s and Fick’s laws

and binary diffusion is applied,

e. Lewis number is unity,

f. individual specific heats are all equal and constant,

g. fuel and oxidizer form products in a single-step exothermic reaction,

h. fuel is completely consumed at the flame with oxidizer in

stoichiometric or excess proportion.

By applying suitable boundary conditions, please derive the expressions for laminar flame velocity and flame thickness. (Simplified solution can be found in most of the combustion textbook, such as the one by S.R. Turns(1996) or the one by I. Glassman (1993 or newer edition).)

Answer 1

1) Mass Conservation equation

Hence,

is the mass flux

Species conservation equation

     

Change in mass flux is balanced by change in mass due to chemical reaction

is the mass fraction of species, is mass diffusivity, is mass produced due to chemical reaction

We balance the stoichiometric equation

1 units of fuel is mixed with units of oxidizer to give of products

Hence,

         

Energy conservation equation

                ----------------------(1)

where is the heat produced due the exothermal equation and is the sum of the heat capacity of individual species

Hence,

      

Since, we can take out as common and club all other individual heat capacities

Now, Lewis number is 1 which means mass diffusivity to heat diffusivity is same or

Putting above relation in eq (1), we get

                                            ----------------------(2)

The flame velocity can be calculated from its mass flux by

                 where S_c is flame velocity

Boundary conditions

Suppose the flame has a thickness

Upstream                                                                                   Downstream

                                                            

                                                                

Integrating equation (2), we get

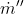
now converting the spatial variable in integration to temperature variable

Now we define the average reaction rate or temperature averaged mass production as

Hence, the energy conservation equation becomes

               --------------------(3)

Above equation has two unknowns, so we have to make an assumption here. We assume that the rate of reaction is slow in upstream than in downstream, then original energy equation becomes

Putting \delta in eq (3)

We know flame speed and

flame thickness

From , we can calculate both flame speed and flame thickness