Question
In: Statistics and Probability
A group of 5 people are to be chosen from a collection of 12 people. Of...
Solutions
Expert Solution
Given
Total number of people = 12
Number of Democrats = 6
Number of Republicans = 4
Number of Independents = 2
Number of people to be chosen = 5
A)
The possible number of committes


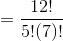
= 792 ways
B)
The possible number of committes consist of 2 Democrats, 2 Republicans, and 1 Independent



= 180 ways
C)
From above,
The possible number of committes chosen ( chosen 5 people from a collection of 12 people)= 792 ways
The possible number of committes consist of 2 Democrats, 2 Republicans, and 1 Independent = 180 ways
Now,
the probability that the committee consists of 2 Democrats, 2 Republicans, and 1 Independent = 180 / 792 = 0.2273
D)
Possible Committee:
i) 4 Democrats, 1 Republicans
ii) 4 Democrats, 1 Independent
iii) 5 Democrats
Now,
The possible number of committees that consist of at least 4 Democrats



= 96 ways
Related Solutions
A team of size m has to be chosen from a group of n people (m...
A committee of 4 is chosen at random from a group of 7 women 5 men....
Suppose that a committee of 12 people is selected in a random manner from a group...
Find the probability that a randomly chosen group of 9 people have no birthdays in common,...
A committee of 2 women and 6 men is to be chosen randomly from a group...
5 people from group are given an ID ranging from 1-9 independent from each other's ID....
John measured the total playing time of 35 randomly chosen CDs from his very large collection...
In a group of 12 astronauts, 5 of them are experts in exobiology. Out of the...
In a group of 12 astronauts, 5 of them are experts in exobiology. Out of the...
Determine the probability that in a group of 5 people, at least two share the same...
- Starting with a 30% (w/w) solution of hydrogen peroxide, how many mL would you need to...
- An object with a density of 761.0 kg/m3 and a mass of 1399.0 kg is thrown...
- British Columbia Lumber has a raw lumber division and a finished lumber division. The variable costs...
- Discussion 1 – due 23 February 2020, 11:55 PM ECT (5% of coursework marks) Provide Sue...
- Describe how at least one of the laws of thermodynamics relates to your room, or the...
- Instruction: The table consists of information about 2 competing investments. ...
- This question is about concentration measurements and its effect on E and K. Cu/Cu^+2 // Ag/Ag^+2...
 orchestra answered 3 years ago
orchestra answered 3 years ago