Question

The following data gives the number of hours 10 students spent studying and their corresponding grades on their midterm exams.

Hours Spent Studying	0	0.5	1	2	2.5	3	4	4.5	5	5.5
Midterm Grades	60	63	75	81	84	87	90	93	96	99

Determine if r is statistically significant at the 0.01 level.

Answer 1

Solution:

n = 10

	X	Y	XY	X^2	Y^2
	0	60	0	0	3600
	0.5	63	31.5	0.25	3969
	1	75	75	1	5625
	2	81	162	4	6561
	2.5	84	210	6.25	7056
	3	87	261	9	7569
	4	90	360	16	8100
	4.5	93	418.5	20.25	8649
	5	96	480	25	9216
	5.5	99	544.5	30.25	9801
Sum	28	828	2542.50	112	70146

Putting values , we get

r = 0.9703

Now , we have to test the significance of correlation coefficient.

Use = 0.01

n = 10

df = n - 2 = 10 - 2 = 8

Using the critical value table for Pearson correlation coefficient, (two tailed )

Critical value are 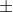 0.765

r = 0.9703

| r | = | 0.9703| = 0.9703

r >  0.765

reject H₀

significance correlation.

YES , there is sufficient evidence to conclude that there is significant  linear correlation between the two​ variables.