Question

The data show the time intervals after an eruption​ (to the next​ eruption) of a certain geyser. Find the regression​ equation, letting the first variable be the independent​ (x) variable. Find the best predicted time of the interval after an eruption given that the current eruption has a height of 126 feet. Use a significance level of 0.05.

Height (ft)   Interval after (min)
84   76
122   77
78   67
108   87
73   61
105   77
122   87
78   70

What is the regression​ equation?

What is the best predicted time for the interval after an eruption that is 126 feet​ high?

Answer 1

Solution:

From given data , we prepare a table.

X	Y	XY	X^2	Y^2
84	76	6384	7056	5776
122	77	9394	14884	5929
78	67	5226	6084	4489
108	87	9396	11664	7569
73	61	4453	5329	3721
105	77	8085	11025	5929
122	87	10614	14884	7569
78	70	5460	6084	4900

n	8
sum(XY)	59012.00
sum(X)	770.00
sum(Y)	602.00
sum(X^2)	77010.00
sum(Y^2)	45882.00
b	0.3691
a	39.7230

Now ,

Slope of the regression line is

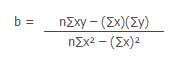
b = 0.3691

Now , y intercept of the line is

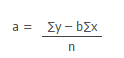
a = 39.7230

The equation of the regression line is

= a + bx

Answer: = 39.7230 +0.3691X

For x = 126 , find the predicted value of y .

Put x = 126 in the regression line equation.

= a + bx

= 39.7230 + (0.3691 * 126)

Answer: y = 86.2296