Question

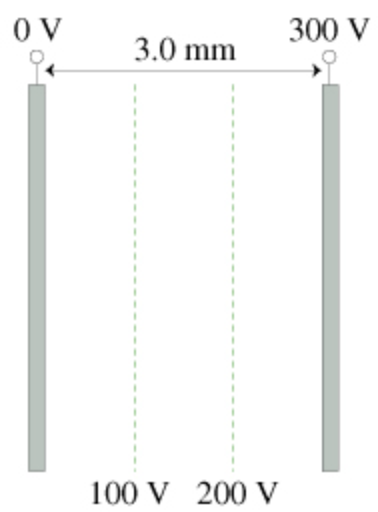
1. In the figure, which capacitor plate, left or right, is the positive plate?

2. What is the electric field strength inside the capacitor?

3. What is the potential energy of a proton at the midpoint of the capacitor?

 

Answer 1

Concepts and reason

The concepts used to solve the problem are the electric fields due to potential and potential energy. Initially, determine which plate is positive by finding the higher potential plate. Then, use the expression of an electric field to find the electric field inside the capacitor. Then, use the expression of potential energy to find the potential energy at the capacitor's mid-point.

Fundamentals

 

The electric field can be given as follows:

\(E=\frac{\Delta V}{\Delta x}\)

Here, \(\mathrm{E}\) is the electric field, \(\Delta V\) is the change in potential, and \(\Delta x\) is the distance between both potentials. The electric potential energy of a charge at potential \(\mathrm{V}\) can be determined as follows:

\(U=q V\)

Here, \(\cup\) is the electric potential, and \(q\) is the charge present at the potential \(V\).

 

(1) The plate having higher potential will be called a positive plate. The plate with \(300 \mathrm{~V}\) is at higher potential as compared to the plate with \(0 \mathrm{~V}\), such that the plate with \(300 \mathrm{~V}\) is called as a positive plate. Thus, the right plate is positive.

Part 1 The right plate is the positive plate.

The value of its potential determines the positivity of a plate compared to the other plate. If the first plate has a 0 V potential and the second plate has a -8 potential, then the first plate is positive.

 

(2) The electric field can be given as follows:

\(E=\frac{\Delta V}{\Delta x}\)

Here, \(\mathrm{E}\) is the electric field, \(\Delta V \Delta x \Delta V\) is the change in potential, and \(\Delta x\) is the distance between both potentials. The change in potential in case of this capacitor is as follows:

\(\Delta V=V_{\mathrm{H}}-V_{\mathrm{L}}\)

Here, \(V_{\mathrm{H}}\) is the higher potential and \(V_{\mathrm{L}}\) is the lower potential. Substitute \(300 \mathrm{~V}\) for \(V_{\mathrm{H}}\) and \(0 \mathrm{~V}\) for \(V_{\mathrm{L}}\) in the above expression.

$$ \begin{aligned} \Delta V &=300 \mathrm{~V}-0 \mathrm{~V} \\ &=300 \mathrm{~V} \end{aligned} $$

Substitute \(300 \mathrm{~V}\) for \(\Delta V\) and \(3.0 \mathrm{~mm}\) for \(\Delta x\) in the expression \(E=\frac{\Delta V}{\Delta x}\)

$$ E=\frac{300 \mathrm{~V}}{3.0 \mathrm{~mm}}\left(\frac{1 \mathrm{~mm}}{10^{-3} \mathrm{~m}}\right) $$

\(=1.0 \times 10^{5} \mathrm{~V} / \mathrm{m}\)

Part 2 The electric field strength inside the capacitor is \(1.0 \times 10^{5} \mathrm{~V} / \mathrm{m}\)

The direction of the electric field inside the capacitor is from higher potential to the lower potential. Thus, the direction of electric field is from plate with 300 V potential to the plate with 0 V potential.

 

(3) The electric potential energy of a charge at potential \(\mathrm{V}\) can be determined as follows:

\(U=q V\)

Here, \(U\) is the electric potential and \(q\) is the charge present at the potential \(V\). The potential at the midpoint of the capacitor lies between potentials \(100 \mathrm{~V}\) and \(200 \mathrm{~V}\). thus, the potential at the midpoint can be considered as \(150 \mathrm{~V}\). Substitute \(150 \mathrm{~V}\) for \(\mathrm{V}\) and \(1.6 \times 10^{-19} \mathrm{C}\) for \(\mathrm{q}\) in the above expression.

$$ \begin{array}{c} U=\left(1.6 \times 10^{-19} \mathrm{C}\right)(150 \mathrm{~V}) \\ =2.4 \times 10^{-17} \mathrm{~J} \end{array} $$

Part 3 The potential energy is \(2.4 \times 10^{-17} \mathrm{~J}\).

The capacitor's midpoint is at half of the total separation between the two plates of the capacitor. Here, the capacitor has a 3 mm separation. Thus, the midpoint lies at a separation of 1.5 mm.