Question

The purpose of this assignment is to calculate and interpret an ANOVA table. For this assignment, use IBM SPSS Statistics.

Part 1:

Using the "Example Dataset," assess this statement using ANOVA: "People with different levels of education exercise for different amounts of time during the week."

Select and conduct the appropriate ANOVA test to assess the statement. Export the ANOVA table to a Word document.

Part 2:

In 250-500 words, discuss the following regarding the use of ANOVA.

1. Describe when the use of ANOVA is more appropriate than the use of a t-test.
2. Describe which ANOVA test you used and why.
3. Interpret the results by (a) stating the reason the study or test was done; (b) presenting the main results, including explaining the within and between subjects variation and the F-ratio from the ANOVA table; (c) explaining what the results mean, including discussing whether there is a statistically significant difference between education groups and amount of exercise; and (d) making suggestions for future research.

   Sex	Education_Level***	Minutes_Exercise
   Female	2	90
   Male	2	50
   Female	3	65
   Male	1	20
   Female	1	50
   Female	2	25
   Female	3	110
   Male	1	50
   Female	2	40
   Male	2	80
   Female	3	120
   Male	1	80
   Female	1	60
   Male	3	150
   Male	2	75
   Male	1	80
   Female	2	110
   Male	3	80
   Male	2	100
   Female	1	0
   Female	2	50
   Female	3	200
   Male	2	60
   Male	1	65
   Female	1	40
   Male	3	65
   Female	3	70
   Female	1	45
   Male	3	75
   Male	3	50

Answer 1

Solution:

Part 1:

Null hypothesis: There is a no statistically significant difference between education groups and amount of exercise

Alternative hypothesis: There is a statistically significant difference between education groups and amount of exercise

Results are summarized below:

Part 1:

Null hypothesis: There is a no statistically significant difference between education groups and amount of exercise

Alternative hypothesis: There is a statistically significant difference between education groups and amount of exercise

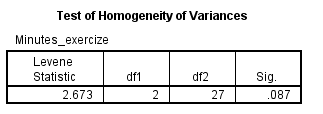
We can observe from table p-value is greater than level of significance 0.05 therefore; we do not reject the null hypothesis of equal variances. Assumption of homogeneity of variances satisfied for performing ANOVA.

P-value is less than level of significance 0.05 therefore we reject the null hypothesis and conclude that there is statistically significant difference between three education level and amount of exercise.

Part 2:

ANOVA test is used when we want to compare more than two or more unrelated groups, whereas two sample t-test is used when we want compare two independent groups.

We have used here, One way ANOVA because researcher wants to check whether there is a statistically significant difference between education groups and amount of exercise. Here we have only one factor or independent variable. A one-way ANOVA only involves one factor or independent variable, whereas there are two independent variables in a two-way ANOVA. ... In a one-way ANOVA, the one factor or independent variable analyzed has three or more categorical groups. A two-way ANOVA instead compares multiple groups of two factors

In given Problem, We want to analyze is there statistically significant difference between three different education level considering amount of exercise. Amount of exercise differs between three education levels or not.

From the ANOVA table we can see between group variability is low compared to within group variability. F-ratio is 5.21 and table value of F at 0.05 for DF1=2 and DF2=29 is 3.32 i.e F-ratio is greater than F-table value therefore; we reject the null hypothesis of equal groups.

From the above results we can reveal that amount of exercise differs between three education levels.

We can study with more data is amount of exercise differs between Males and females with different education levels.