Question
In: Statistics and Probability
At Case Western Reserve University, 46.6% of the student body are undergraduates and 53.4% of those...
At Case Western Reserve University, 46.6% of the student body are undergraduates and 53.4% of those enrolled are graduate students. 60.7% of undergraduate students participate in extracurricular activities, while only 32.1% of graduate students participate in extracurricular activities. A student who participates in extracurricular activities is randomly selected. What is the probability that the student is an undergraduate? Use Baye’s theorem to solve this problem. (Write your answer as a percent rounded to 1 decimal place.)
Solutions
Expert Solution
Solution:
Given: 46.6% of the student body are undergraduates and 53.4% of those enrolled are graduate students.
Let UG = Student is Undergraduates and G = Student is Graduates
Thus we have:
P(UG) = 0.466
P(G) =0.534
60.7% of undergraduate students participate in extracurricular activities.
Let EA = Students participate in Extracurricular Activities.
thus
P(EA | UG) = 0.607
32.1% of graduate students participate in extracurricular activities.
P(EA | G) = 0.321
We have to find:
P( the student is an undergraduate given that student participates in extracurricular activities ) =..........?
P(UG | EA) =..........?
Using Baye’s theorem of probability we get:
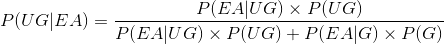
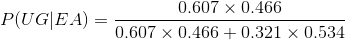
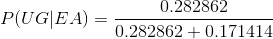
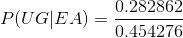
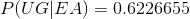
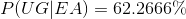

Related Solutions
A Positive Revolution in Change: Appreciative Inquiry David L. Cooperrider Case Western Reserve University and Diana...
at a large University the graduation rate for the general student body was reported to be...
A survey at Evashevski University showed that 75% of the student body believes that the school...
Chapter 15 The University Bookstore Student Computer Purchase Program Case page 762 The University Bookstore Student...
The student body of a large university consists of 30% Business majors. A random sample of...
[6] Sixty percent of the student body at the University of British Columbia is from British...
An Office of Admission document at a certain university claims that 55.3% of their undergraduates are...
In 2015, the student body of a Lock Haven University consisted of 30% freshmen, 24% sophomores,...
***URGENT*** TEST 1. At one large university, freshmen account for the 40% of the student body,...
A study conducted at a major university indicates that the tendency among undergraduates to seek the...
- Starting with a 30% (w/w) solution of hydrogen peroxide, how many mL would you need to...
- An object with a density of 761.0 kg/m3 and a mass of 1399.0 kg is thrown...
- British Columbia Lumber has a raw lumber division and a finished lumber division. The variable costs...
- Discussion 1 – due 23 February 2020, 11:55 PM ECT (5% of coursework marks) Provide Sue...
- Describe how at least one of the laws of thermodynamics relates to your room, or the...
- Instruction: The table consists of information about 2 competing investments. ...
- This question is about concentration measurements and its effect on E and K. Cu/Cu^+2 // Ag/Ag^+2...
 orchestra answered 3 years ago
orchestra answered 3 years ago