Question

a) Describe the specific energy.

b) In the rectangular duct, analyze the flow (Q) as a function of depth (y) for constant specific energy (E = E0).

c) Define critical depth (yc) and critical speed (Vc) for this case.

d) Plot the flow (Q) - depth (y) graph and show the regimes of the flow.

Answer 1

(a)

Specific energy of a liquid in an open channel is the total mechanical energy of the flow relative to the bottom of the channel.

As we can see from the image, the specific energy is the energy compared to the bottom of the flow.

(b)

The below graph shows the relation between depth y and specific energy E for different flows, q₁, q₂, and q₃.

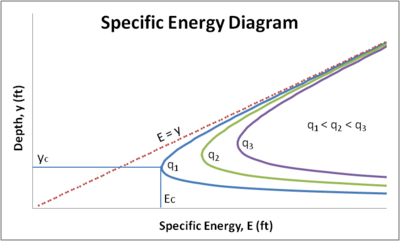
At a constant specific energy, it is clear that flow increases as depth increases.

(c)

When the flow reaches a minimum value of specific energy, this point is called the critical point. The value of depth at this particular point is called the critical depth. The specific energy at this point is termed as the critical energy, and the velocity of the flow at this particular point is called the critical velocity.

In the above graph, the critical point of the flow q₁ is given at the values of energy and depth are given as E_c and y_c respectively.

(d)

The same graph can be used for plotting the flow vs depth graph. The different flows are given with the q₁, q₂, and q₃ curves.