Question

In: Math

To navigate on Lake Latte (fed by the Decaf and the Vanilla Rivers) at least two...

To navigate on Lake Latte (fed by the Decaf and the Vanilla Rivers) at least two of the three
radio navigation beacons must be working. If the probability that a beacon is working is
p and the operational status of each station is independent of the other two, what is the
probability of being able to navigate on the lake? What is the probability that beacon #2 is
working if navigation on the lake is possible?

Solutions

Expert Solution

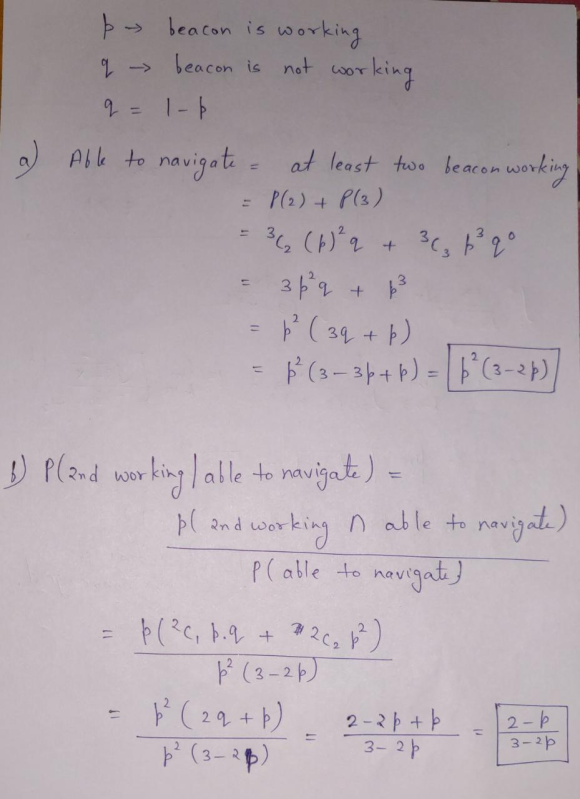
part b) is a bit tricky.

In that when we find that P(2nd working and able to navigate) means P(2nd working, one more beacon working) + P(2nd working, 2 more beacons working)

the outer 'p' is showing that 2nd working and in the bracket we got 2C1 which is selecting 1 beacon which is working, p is probability of that beacon to work and q is probability that another beacon not working. and same way 2C2 is showing both beacons working and p^2 is probability of those to work.

I hope it will be easy to understand. Please comment if anything is not clear.

milcah answered 1 day ago
Next > < Previous

Related Solutions

A solution of hydrochloric acid accidentally leaks into a spring fed freshwater lake, which in turn...
A solution of hydrochloric acid accidentally leaks into a spring fed freshwater lake, which in turn feeds a single stream. the acid leaks into the lake at a rate of 2 gallons per hour and has a concentration if 3 pounds per gallon. The lake initially contains 4000 gallons of water. Water flows into the lake from the spring at a rate of 100 gallons per hour and fluid flows out of the lake into the stream at a rate...
Consider a 10x108 m3 lake fed by a polluted stream having a flow rate of 2.0...
Consider a 10x108 m3 lake fed by a polluted stream having a flow rate of 2.0 m3 /s and pollutant concentration equal to 10.0 mg/L. There is also a sewage outfall that discharges 0.5 m3 /s of wastewater that has a pollutant concentration of 100 mg/L into the lake. The stream and sewage pollutants have a decay rate coefficient of 0.20 day-1 (first order). Under these flow conditions the lake remains the same volume (e.g. a stream enters and exits...
Rio Coffee Shoppe sells two coffee drinks—a regular coffee and a latte. The two drinks have...
Rio Coffee Shoppe sells two coffee drinks—a regular coffee and a latte. The two drinks have the following prices and cost characteristics: Regular Coffee Latte Sales price (per cup) $ 1.60 $ 2.90 Variable costs (per cup) 0.80 1.70 The monthly fixed costs at Rio are $5,984. Based on experience, the manager at Rio knows that the store sells 80 percent regular coffee and 20 percent lattes. Required: How many cups of regular coffee and lattes must Rio sell every...
If Fed wants to add at least $50 billion into the market, what would it do?...
If Fed wants to add at least $50 billion into the market, what would it do? (Theoretically, there are more than one way. Though you will get credit if you choose other ways, I expect you to give the most practical one)
How are rivers structured differently than oceans or lakes? Give two abiotic conditions this difference is...
How are rivers structured differently than oceans or lakes? Give two abiotic conditions this difference is likely to affect and explain how?
You manage an ice cream factory that makes two flavors: Creamy Vanilla and Continental Mocha. Into...
You manage an ice cream factory that makes two flavors: Creamy Vanilla and Continental Mocha. Into each quart of Creamy Vanilla go 2 eggs and 3 cups of cream. Into each quart of Continental Mocha go 1 egg and 3 cups of cream. You have in stock 500 eggs and 900 cups of cream. You make a profit of $3 on each quart of Creamy Vanilla and $2 on each quart of Continental Mocha. How many quarts of each flavor...
You manage an ice cream factory that makes two flavors: Creamy Vanilla and Continental Mocha. Into...
You manage an ice cream factory that makes two flavors: Creamy Vanilla and Continental Mocha. Into each quart of Creamy Vanilla go 2 eggs and 3 cups of cream. Into each quart of Continental Mocha go 1 egg and 3 cups of cream. You have in stock 400 eggs and 750 cups of cream. You make a profit of $3 on each quart of Creamy Vanilla and $2 on each quart of Continental Mocha. How many quarts of each flavor...
Which headline would be LEAST likely to be studied in macroeconomics? he Fed Raises Interest Rates...
Which headline would be LEAST likely to be studied in macroeconomics? he Fed Raises Interest Rates Economic, Growth Rises Slow and Steady GM Expands Car Sales in China Unemployment Falls to a 7-Year Low
Can the FED control the money supply? Please answer in at least 3 paragraphs Your paper...
Can the FED control the money supply? Please answer in at least 3 paragraphs Your paper should include the following: Introduction –what is the question you are addressing? What are the main issues? Give the reader a quick summary of what you will explain. Body Brief discussion of the text book view—exogenously controlled money (via the mm relationship). What does real world evidence suggest about the central bank’s ability to control money? Conclusion – answer the question based on your...
Select at least two of the following types of organizations and describe at least two types...
Select at least two of the following types of organizations and describe at least two types of standard costs they might use: Cleaning service Insurance agent/agency Physician’s office Lube and tire shop Additionally, explain the types of direct and overhead variances each of your selected organizations might encounter. Provide an example of each.
ADVERTISEMENT
Subjects
ADVERTISEMENT
Latest Questions
ADVERTISEMENT