Question

A flywheel with a diameter of 6.5 m is rotating at an angular speed of 150 rev/min. After 95 s the flywheel rotates with angular speed to 375 rev/min. Find the angular displacement produced by the flywheel during 25 s interval.

A.

876 rad

B.

678 rad

C.

687 rad

D.

867 rad

Answer 1

8.

Let angular acceleration of flywheel =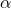

By using first rotational kinematics law,

wf - wi = 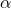*t

here, wf = final angular speed = 375 rev/min = 375*(2*pi/60) = 39.3 rad/s

wi = initial angular pseed = 150 rev/min = 150*(2*pi/60) = 15.7 rad/s

t = time interval = 95 s

then, 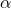= (39.3 - 15.7)/95 = 0.25 rad/s^2

Now, by using second rotational kinematics law,

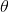= angula displacement = wi*t + 0.5*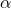*t^2

here, t = 25 s

then, 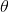= 15.7*25 + 0.5*0.25*25^2

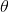= 470 rad

"There must be an typing error where it is mentioned that "after 95 s the flywheel rotates", if we use 95 sec then there will be no answer. But if we use 25 sec in place of 95 sec, then answer will be 687 rad. Please ask this issue with your TA or professor before giving negative rating"

Now, if we use 25 s in place of 95 s,

Let angular acceleration of flywheel =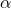

By using first rotational kinematics law,

wf - wi = 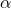*t

here, wf = final angular speed = 375 rev/min = 375*(2*pi/60) = 39.3 rad/s

wi = initial angular pseed = 150 rev/min = 150*(2*pi/60) = 15.7 rad/s

t = time interval = 25 s

then, 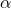= (39.3 - 15.7)/25 = 0.944 rad/s^2

Now, by using second rotational kinematics law,

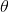= angula displacement = wi*t + 0.5*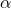*t^2

here, t = 25 s

then, 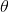= 15.7*25 + 0.5*0.944*25^2

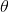= 687 rad

then, correct option is C.

"Let me know if you have any query."