Question

A planet has the same density as Earth but has a surface gravitational acceleration of nine times that of

the Earth (a person would instantly black out if placed on the surface). What is the escape velocity from the planet? If

the planet was in an orbit of 1 AU from a 1 M⊙ star, what is the maximum distance that a moon could orbit the planet?

Answer 1

Escape velocity is the minimum speed an object must possess to escape the planet's gravitational field. We can easily calculate the expression for escape velocity( you must have done it in class). Let us say that the object is initially at a distance r from the center of the planet. If the object is on the surface, r=R, the planet's radius. So, it has potential energy where M and m are masses of planet and object respectively. Let us say it has velocity v initially. SO, the kinetic energy is   . Let us say it escapes the gravitational field eventually. SO potential energy finally is 0. . Since we want to find the minimum initial velocity such that this happens, let us set final velocity to 0. SO, . By conservation of energy, .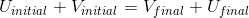

So, and rearranging we get the escape velocity

This makes use of the mass and radius of the planet which we don't know.

So, we can write where rho is the density and is the volume of the planet (considering it as a perfect sphere)

Also, gravitational accleration of a planet is given by which gives

We have . Replacing M,

Substitute the expression for R

So, given that the densities of the two planets is same, the escape velocity depends directly depends on the gravitational acceleration. Since g of the planet is nine times that of earth, the escape velocity is 9 times earth's escape velocity, Since earth's escape velocity is 11,186m/s, the planet's escape velocity is 100,674m/s

The maximum distance that a moon could orbit a planet is called the radius of its sphere of influence. Within this sphere, the influence of the planet exceeds the influence of the star on the moon. This radius is given by where a is the orbital radius of the planet and M_s is the mass of the star.

We know that the mass of the planet is

Everything here is a constant except density and gravitational acceleration and since density is the same as earth and density is 9 times, the mass of the planet is times the mass of earth.

We know that the mass of earth is in terms of solar masses. SO, the mass of the given planet is

Therefore, the radius of influence is

The moon could orbit at a maximum distance of 0.0863AU