Question

In: Physics

Consider a uniform density sphere of mass M and radius R in hydrostatic equilibrium with zero...

Consider a uniform density sphere of mass M and radius R in hydrostatic equilibrium with zero surface pressure. Derive expressions for the pressure P(r) and the gravitational potential phi(r) in terms of r, M, R, G and constants.

Solutions

Expert Solution

Let the uniform density be .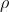

Consider a thin shell of thickness dr at a distance r from the centre of the star. Mass of the shell dm will be,

Then the Gravitational force on the shell will be due to the interior mass of the star, Therfore we can write the gravitational force as.

The mass of the interior portion of the star can be written as,

Substituting we get,

This inward force is balanced by the outward pressure of the gas. The outward pressure will be

P(r) - P(r+dr) (Since P(r+dr) < P(r), The surface pressure is 0) (Here the sign followed is inward force is +ve, as in the case for gravitational force and outward force would be -ve)

Therfore we can write,

Now, we have to integrate both sides from R to distance r (since pressure at surface is 0), so as to find P(r)

Substituting value of density we can write,

Here, R is the radius of the star.

Gravitational potential is defined as work done per unit mass of the particle to bring a particle of mass m from infinity to the point at a distance of r, it can be evaluated by,

The gravitational potential for distance r>R is given by,

genius_generous answered 2 years ago
Next > < Previous

Related Solutions

Consider a thin uniform disk of mass M and radius R. A mass m is located...
Consider a thin uniform disk of mass M and radius R. A mass m is located along the axis of the disk at a distance z from the center of the disk. The gravitational force on the mass m (in terms of m, M, R, G, and z) is
Consider a wire of uniform density and mass M shaped into a quarter circle of radius...
Consider a wire of uniform density and mass M shaped into a quarter circle of radius R.The wire is located in the xy plane, with the origin at the focus of the quarter circle (i.e at the center of the circle of which the wire is part). Choose the x axis parallel to the line that connects the endpoints of the wire, so that the y-z plane is a plane of reflection symmetry for the wire. This means that the...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is placed in a large bowl containing water. It floats and the level of the water in the dish is L. Given the information below, determine the possible effects on the water level L, (R-Rises, F-Falls, U-Unchanged), when that sphere is replaced by a new solid sphere of uniform density. Read it to me R F U R or U F or U R or...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is placed in a large bowl containing water. It floats and the level of the water in the dish is L. Given the information below, determine the possible effects on the water level L, (R-Rises, F-Falls, U-Unchanged), when that sphere is replaced by a new solid sphere of uniform density. The new sphere has mass M = M0 and radius R > R0 The new...
A small solid sphere of mass M0, of radius R0, and of uniform density ?0 is...
A small solid sphere of mass M0, of radius R0, and of uniform density ?0 is placed in a large bowl containing water. It floats and the level of the water in the dish is L. Given the information below, determine the possible effects on the water level L, (R-Rises, F-Falls, U-Unchanged), when that sphere is replaced by a new solid sphere of uniform density. Read it to me R F U R or U F or U R or...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is placed in a large bowl containing water. It floats and the level of the water in the dish is L. Given the information below, determine the possible effects on the water level L, (R-Rises, F-Falls, U-Unchanged), when that sphere is replaced by a new solid sphere of uniform density. The new sphere has mass M = M0 and density ρ < ρ0 The new...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is placed in a large bowl containing water. It floats and the level of the water in the dish is L. Given the information below, determine the possible effects on the water level L, (R-Rises, F-Falls, U-Unchanged), when that sphere is replaced by a new solid sphere of uniform density. R F U R or U F or U R or F or U  The new...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is...
A small solid sphere of mass M0, of radius R0, and of uniform density ρ0 is placed in a large bowl containing water. It floats and the level of the water in the dish is L. Given the information below, determine the possible effects on the water level L, (R-Rises, F-Falls, U-Unchanged), when that sphere is replaced by a new solid sphere of uniform density. Read it to me R F U R or U F or U R or...
A hollow sphere of mass M and radius R is hit by a pendulum of mass...
A hollow sphere of mass M and radius R is hit by a pendulum of mass m and length L that is raised at an angle θ. After the collision the pendulum comes to a stop and the sphere rolls forward into a spring with stiffness k on an incline of ϕ. Find an expression for how far ∆x the spring compresses along the incline. Use only m, L, M, R, θ, ϕ, k and appropriate constants.
A non conducting sphere of radius R and uniform volume charge density is rotating with angular...
A non conducting sphere of radius R and uniform volume charge density is rotating with angular velocity, Omega. Assuming the center of the sphere is at the origin of the coordinate system, a) what is the magnitude and direction of the resulting magnetic field on the z axis for any arbitrary z distance away from the origin when z > R? b) same question as part a) but for z < R? Omega of the rotating sphere on the extra...
ADVERTISEMENT
Subjects
ADVERTISEMENT
Latest Questions
ADVERTISEMENT