Question
In: Statistics and Probability
A survey of the mean number of cents off that coupons give was conducted by randomly...
A survey of the mean number of cents off that coupons give was conducted by randomly surveying one coupon per page from the coupon sections of a recent Rockford newspaper. The following data were collected: 20¢; 75¢; 50¢; 75¢; 30¢; 55¢; 10¢; 40¢; 30¢; 55¢; $1.50; 40¢; 65¢; 40¢. Assume the underlying distribution is approximately normal. Construct a 95% confidence interval for the population mean worth of coupons. Find the lower limit of the confidence interval. Enter it rounded to the nearest cent (2 decimal places)
Solutions
Expert Solution
Solution:
| x | x2 |
| 20 | 400 |
| 75 | 5625 |
| 50 | 2500 |
| 75 | 5625 |
| 30 | 900 |
| 55 | 3025 |
| 10 | 100 |
| 40 | 1600 |
| 30 | 900 |
| 55 | 3025 |
| 1.5 | 2.25 |
| 40 | 1600 |
| 65 | 4225 |
| 40 | 1600 |
| ∑x=586.5 | ∑x2=31127.25 |
The sample mean is 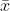
Mean 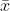 = (
= ( x
/ n) )
x
/ n) )
=20+75+50+75+30+55+10+40+30+55+1.5+40+65+40 /14
=586.5/14
=41.8929
Mean 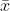 = 41.89
= 41.89
The sample standard is S
S =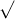 (
(
 x2 ) - ((
x2 ) - ((  x)2 / n ) n -1
x)2 / n ) n -1
=√31127.25-(586.5)21413
=√31127.25-24570.1607/13
=√6557.0893/13
=√504.3915
=22.4587
Degrees of freedom = df = n - 1 = 14 - 1 = 13
At 95% confidence level the t is ,
 =
1 - 95% = 1 - 0.95 = 0.05
=
1 - 95% = 1 - 0.95 = 0.05
 / 2 = 0.05 / 2 = 0.025
/ 2 = 0.05 / 2 = 0.025
t /2,df = t0.025,13 =2.160
/2,df = t0.025,13 =2.160
Margin of error = E = t /2,df
* (s /
/2,df
* (s /n)
= 2.160 * (22.46 /
14)
= 12.97
Margin of error = 12.97
The 95% confidence interval estimate of the population mean is,
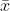 - E
- E
41.89 - 12.97
= 28.92
The lower limit = 28.92
Related Solutions
A survey of the mean number of cents off that coupons give was conducted by randomly...
A survey of the mean number of cents off that coupons give was conducted by randomly...
A survey of the mean number of cents off that coupons give was conducted by randomly...
A survey of the mean number of cents off that coupons give was conducted by randomly...
A certain survey is conducted by choosing randomly 10 students and asked them about the number...
A survey was conducted to estimate the mean number of books (denoted by µ) each university...
A survey of 36 randomly selected students who dropped a course was conducted at a college....
A survey was conducted to determine whether a household's income was associated with the number of...
The number of unemployed is calculated by: a large monthly survey of households conducted for the...
A hospital conducted a survey to estimate the mean length of stay at the hospital. a...
- Write the code for binary min heap in c++ .
- Starting with a 30% (w/w) solution of hydrogen peroxide, how many mL would you need to...
- An object with a density of 761.0 kg/m3 and a mass of 1399.0 kg is thrown...
- British Columbia Lumber has a raw lumber division and a finished lumber division. The variable costs...
- Discussion 1 – due 23 February 2020, 11:55 PM ECT (5% of coursework marks) Provide Sue...
- Describe how at least one of the laws of thermodynamics relates to your room, or the...
- Instruction: The table consists of information about 2 competing investments. ...
 orchestra answered 2 years ago
orchestra answered 2 years ago