Question

(PLEASE SHOW WORK) - no financial calculator - no excel

2. David Hoffman purchases a bond with $1,000 principal, 20-year maturity, and 8% coupon (annual payments). Yields on comparable bonds are 10%.   Bob expects that two years from now, yields on comparable bonds will have declined to 9%. Find his expected yield, assuming the bond is sold in two years.

3. Calculate the duration of a $1,000, eight-year zero coupon bond using annual compounding and a current market rate of 7 per cent.

4. Calculate the duration for a $1000, 4-year bond with a 4.5% annual coupon, currently selling at par. Use the duration to estimate the percentage change in the bond’s price for a decrease in the market interest rate to 3.5%.

Answer 1

2.

Given information:

Coupon rate = 8%

Interest rate ( rate) = 10%

Years (nper) = 20

Future value (FV) = $1,000

Coupon payment (pmt) = coupon rate * FV

= 8% * $1,000 = $80

Calculations are:

Present value (PV at time 0) = $829.73

By using excel formula:

=PV(rate,nper,pmt,fv,type)

=PV(10%,20,80,1000,0)

$829.73

After 2 years interest rate = 9%, years = 18,

Then the present value = $912.44

By using excel formula

=PV(rate,nper,pmt,fv,type)

=PV(9%,18,80,1000,0)

$912.44

Expect yield = 14%

By using excel formula

=rate(nper, pmt, PV, FV, type)

=rate(2,80,-829.73,913.44,0)

14%

3.

Duration = 8 years

In zero coupon bond, the duration is Bond's time to maturity.

4.

Given information:

Coupon rate = 4.5%

Years = 4

Future value = $1,000

Coupon payment = coupon rate * future value

= 4.5% * $1,000

= $45

Present value of cash flow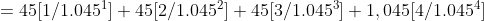

= $3748.97

Duration = present value of cash flow/future value

= $3,748.97/$1,000

=3.75 Years.