Question
In: Finance
There are two parts to this question first part: Consider a production facility, where the present...
There are two parts to this question first part:
Consider a production facility, where the present value of expected future cash inflows from production, V = 80, may fluctuate in line with the random fluctuation in demand (u = 1.4, d = 0.71 per period and the risk-free rate, r = 5%). Suppose management has the option in two years, to contract to half the scale and half the value of the project (c = 50%), and recover $40m (Rc = $40m). Thus, in year 2 management has the flexibility either to maintain the same scale of operations (i.e., receive project value, V, at no extra cost) or contract the scale of operations and receive the recovery amount, whichever is highest. What are the pay-offs of this option at the end nodes (thus in the different states after 2 periods)?
The payoffs, F, of the option in the end note states are respectively: F = 0 , F = 0, F = 20
The payoffs, F, of the option in the end note states are respectively: F = 0 , F = 0, F = 14
The payoffs, F, of the option in the end note states are respectively: F = 196 , F = 100, F = 51
The payoffs, F, of the option in the end note states are respectively: F = 157 , F = 80, F = 41
Second part:
Consider again the production facility (from question above). Again, suppose that management has the option in two years, to halve the scale and the value of the project and recover some value. Thus, in year 2 management has the flexibility either to maintain the same scale of operations or contract the scale of operations, whichever is highest.
For this question, assume the end node pay-offs are 0, 20, 50. Calculate the option value by discounting with the risk neutral probability of 0.5 and a risk free rate of 5%. What is the option value?
Solutions
Expert Solution
Part 1:
This is a classic binomial model problem. Let us start with a few notations.
 represents the present value of expected future cash inflows from
production V in case it moves up in both the
periods at the end of the second period. Hence,
represents the present value of expected future cash inflows from
production V in case it moves up in both the
periods at the end of the second period. Hence,

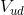 represents the present value of expected future cash inflows from
production V in case it moves up in the first
period and moves down in the second period.
represents the present value of expected future cash inflows from
production V in case it moves up in the first
period and moves down in the second period. 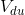 represents the present value of expected future cash inflows from
production V in case it moves down in the first
period and moves up in the second period. In both cases,
represents the present value of expected future cash inflows from
production V in case it moves down in the first
period and moves up in the second period. In both cases,

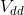 represents the present value of expected future cash inflows from
production V in case it moves up in both the
periods. Hence,
represents the present value of expected future cash inflows from
production V in case it moves up in both the
periods. Hence,

In all the three states at the end of two periods, given the value is more than 40, it doesn't make sense to exercise the option in any of the states.. Hence, the option doesn't hold any value here. F = 0 for all the three states.
Part 2:
In this case, if we assume  and
and  be the option values at the end of two periods in case the value
goes up in both, values goes up in first and down in second, the
value goes down in first and up in second, and it goes down in both
the periods respectively, we can write:
be the option values at the end of two periods in case the value
goes up in both, values goes up in first and down in second, the
value goes down in first and up in second, and it goes down in both
the periods respectively, we can write:

If  and
and  represents the option value in up and down states respectively at
the end of period 1,
represents the option value in up and down states respectively at
the end of period 1,


If  represents the option value today,
represents the option value today,

Hence, the value of the option today is $20.40.
Related Solutions
This question has two parts. Please answer both, thank you! Part A.) On the first day...
Question 1 The binomial formula has two parts. The first part of the binomial formula calculates...
There are two unrelated parts to this question. Part 1 is worth 9 marks and Part...
This problem is about the java programming and contain two parts First part: a word that...
Please note that this assignment consists of two separate parts. The first part gives the cash...
This question has 8 parts. Each of the 8 parts (Part A - Part H) has...
This question has 7 parts. Each of the 7 parts (Part A - Part G) has...
Consider two countries, Spain and Italy, where the only two factors of production are capital and...
A feed manufacturing company is faced with a capacity decision. Their present production facility is running at...
First on the president’s list is an evaluation of where the firm is at the present....
- Your neighbor tells you that her nine-year-old son has been having behavior and academic problems in...
- I can’t get my code to work and/or finish it. Please fix. Below are code instructions...
- Three entrepreneurs plan to open a copy shop. It costs $5000 per year to rent a...
- I understand how electrons initially move into another's vicinity, but nowhere can I find a fathomable...
- The work climate has proven to be an effective measuring stick to successful performance. Tell what...
- 1b. Explain each of the following with an example in two languages of your choice for...
- Zinc sulfide, ZnS, exists in two main crystal forms. The more stable form, zinc blende, is...
 represents the present value of expected future cash inflows from
production V in case it moves up in both the
periods at the end of the second period. Hence,
represents the present value of expected future cash inflows from
production V in case it moves up in both the
periods at the end of the second period. Hence,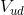 represents the present value of expected future cash inflows from
production V in case it moves up in the first
period and moves down in the second period.
represents the present value of expected future cash inflows from
production V in case it moves up in the first
period and moves down in the second period. 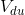 represents the present value of expected future cash inflows from
production V in case it moves down in the first
period and moves up in the second period. In both cases,
represents the present value of expected future cash inflows from
production V in case it moves down in the first
period and moves up in the second period. In both cases,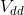 represents the present value of expected future cash inflows from
production V in case it moves up in both the
periods. Hence,
represents the present value of expected future cash inflows from
production V in case it moves up in both the
periods. Hence, jeff jeffy answered 2 years ago
jeff jeffy answered 2 years ago