Question

A company that produces coffee for use in commercial machines monitors the caffeine content in its coffee. The company selects 35 8-oz samples each hour from its production line to analyze. The samples collected one morning between 8:00 - 9:00 am contained on average 96.1 mg of caffeine, with standard deviation 1.2 mg.

1. a) Compute and interpret a 95% confidence interval for mean caffeine content based on the collected data.

2. b) According to production standards, the mean amount of caffeine content per 8 ounces should be no more than 95 mg. An overly high caffeine content indicates that the coffee beans have not been roasted long enough.

   Conduct a formal hypothesis test to investigate whether production standards are being met, based on the observed data. Summarize your findings to the CEO using language accessible to someone who has not taken a statistics course and make a recommendation as to whether an adjustment needs to be made to the bean roasting time.

3. c) A set of samples collected between 10:00 - 11:00 am on the same day has average caffeine content of 95.3 mg, with standard deviation 1.1 mg. Based on observing this data, would you change your recommendation in part c)? Explain your answer.

Answer 1

a)

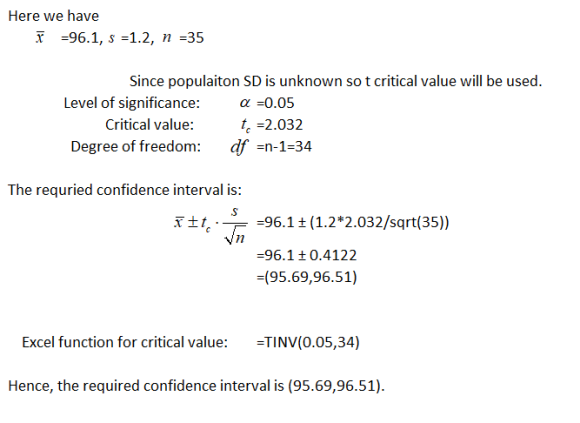
Interpretation: We are 95% confident that true mean caffeine content lie in the above interval.

(b)

Conclusion: There is evidence to conclude that  the mean amount of caffeine content per 8 ounces is more than 95 mg.

That is beans coffee beans have not been roasted long enough.  An adjustment needs to be made to the bean roasting time.

(c)

Conclusion: There is no evidence to conclude that  the mean amount of caffeine content per 8 ounces is more than 95 mg.

That is beans coffee beans have been roasted long enough. No adjustment needs to be made to the bean roasting time.