Question
In: Math
It is estimated that approximately 8.17% Americans are afflicted with diabetes. Suppose that a certain diagnostic...
It is estimated that approximately 8.17% Americans are afflicted with diabetes. Suppose that a certain diagnostic evaluation for diabetes will correctly diagnose 98% of all adults over 40 with diabetes as having the disease and incorrectly diagnoses 3% of all adults over 40 without diabetes as having the disease. a) Find the probability that a randomly selected adult over 40 does not have diabetes, and is diagnosed as having diabetes (such diagnoses are called "false positives"). b) Find the probability that a randomly selected adult of 40 is diagnosed as not having diabetes. c) Find the probability that a randomly selected adult over 40 actually has diabetes, given that he/she is diagnosed as not having diabetes (such diagnoses are called "false negatives"). (Note: it will be helpful to first draw an appropriate tree diagram modeling the situation)
Solutions
Expert Solution
Solution:
Let
TP = Test Positive
TN = Test Negative
D = having diabetes
ND = Not having diabetes
Thus we have:
8.17% Americans are afflicted with diabetes.
That is:
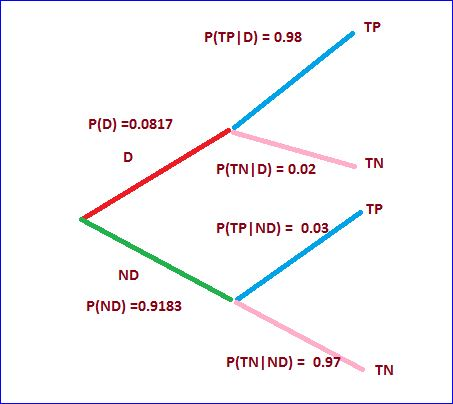
P(D) = 0.0817
P(ND) = 1 - P(D)
P(ND) = 1 - 0.0817
P(ND) = 0.9183
A certain diagnostic evaluation for diabetes will correctly diagnose 98% of all adults over 40 with diabetes as having the disease
P( TP | D) = 0.98
and
incorrectly diagnoses 3% of all adults over 40 without diabetes as having the disease
P( TP | ND)= 0.03
Thus Tree diagram is:
Part a) Find the probability that a randomly selected adult over 40 does not have diabetes, and is diagnosed as having diabetes
P( Does not have diabetes, and is diagnosed as having diabetes) = ........?
P( ND and TP ) =............?
Using: P( TP | ND)= 0.03 and conditional probability formula:
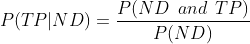
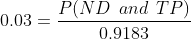
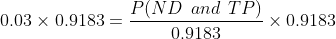


Part b) Find the probability that a randomly selected adult of 40 is diagnosed as not having diabetes.
That is find:
P( TN) = ..........?
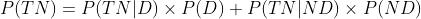
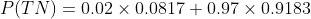
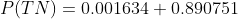

Part c) Find the probability that a randomly selected adult over 40 actually has diabetes, given that he/she is diagnosed as not having diabetes
P(D | TN) =.............?
Using Bayes rule:
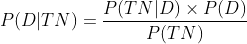
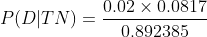
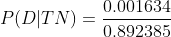
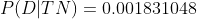
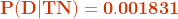
Related Solutions
(1 point) It is estimated that approximately 8.1% of Americans are afflicted with diabetes. Suppose that...
Suppose the lengths of the pregnancies of a certain animal are approximately distributed with a mean...
A certain medical test is known to detect 72% of the people who are afflicted with...
A certain medical test is known to detect 73% of the people who are afflicted with...
A certain medical test is known to detect 50% of the people who are afflicted with...
A certain medical test is known to detect 49% of the people who are afflicted with...
A certain medical test is known to detect 38% of the people who are afflicted with...
Suppose the lengths of the pregnancies of a certain animal are approximately normally distributed with mean...
Suppose the lengths of the pregnancies of a certain animal are approximately normally distributed with mean...
Suppose the lengths of the pregnancies of a certain animal are approximately normally distributed with mean...
- The work climate has proven to be an effective measuring stick to successful performance. Tell what...
- 1b. Explain each of the following with an example in two languages of your choice for...
- Zinc sulfide, ZnS, exists in two main crystal forms. The more stable form, zinc blende, is...
- Assignment # 6: Chain of Custody Roles and Requirements Learning Objectives and Outcomes Describe the requirements...
- What biological, psychological, and social cultural influences affect our experience of pain? How do placebos, distraction,...
- A spherical object has an outside diameter of 60.0cm . Its outer shell is composed of...
- Write the code for binary min heap in c++ .
 milcah answered 9 months ago
milcah answered 9 months ago