Question

Madsen Motors's bonds have 16 years remaining to maturity. Interest is paid annually, they have a $1,000 par value, the coupon interest rate is 12%, and the yield to maturity is 13%. What is the bond's current market price? Round your answer to the nearest cent.

$  

Answer 1

Face value of the bond = FV = $1,000

Interest is paid annually

Annual coupon rate = 12%

Annual coupon payment = Coupon rate x Face Value = 12%*1000 = 120

Time to maturity = 16 years

Yield to maturity = YTM = 13%

Method 1: Uisng ba ii plus calculator

N = 16, I/Y = 13, PMT = 120, FV = 1000

CPT -> PV (Press CPT and then press PV)

We get, PV = -933.9612494

So, the current market price of the bond is $933.96 (rounded to nearest cent)

Answer -> current market price of the bond = 933.96

Method 2: Using Excel

We can use the PV function in Excel to calculate the present value of the bond, as shown below:

=PV(13%,16,120,1000) = -933.9612494

Current market price of the bond = 933.96

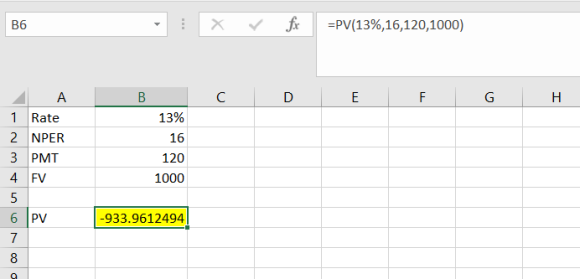
Answer -> Bond's current market price = 933.96

Method 3 - Using Formula

Price of the bond is the sum of the present value of all the future cash flows

Since the bond pays annual interest payments the cash flows of the bond are:

C₁ = C₂ =.......=C₁₅ = 120, C₁₆ = 1120

The present value of these cashflows can be calculated using the formula

PV = C/(1+r)ⁿ

Below table shows the cash flows and the present value of the cash flows

Year	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	16
Cash flow	120	120	120	120	120	120	120	120	120	120	120	120	120	120	120	1120
Present value of Cashflows	106.1947	93.9776	83.16602	73.59825	65.13119	57.63822	51.00728	45.13918	39.94618	35.3506	31.28372	27.68471	24.49974	21.68119	19.18689	158.4758

Price of the bond = 106.1947+93.9776+83.16602+73.59825+65.13119+57.63822+51.00728+45.13918+39.94618+35.3506+31.28372+27.68471+24.49974+21.68119+19.18689+158.4758 = 933.96127

Answer -> Price of the bond = 933.96