Question
In: Economics
A monopolist faces three groups of customers (or three markets) such that: Demand of the high-income...
A monopolist faces three groups of customers (or three markets) such that:
Demand of the high-income group is given by Ph= 24 – 2Qh
Demand of the middle-income group is given by Pm= 20 – 2Qm
Demand of the low-income group is given by Pl= 16 – 2Ql
- If the monopolist does not practice price discrimination (PD) and combines the three markets into one big market, then he charges a single price to everyone. If his marginal cost MC = $14, find the single price Ps, total quantity sold in the big market Qt, and quantity sold to each group alone: Qh, Qm, & Ql.
- Find consumers’ surplus CS for each group or in each market.
- Suppose the monopolist now decides to practice PD. His MC has not changed. Find the three prices charged: Ph, Pm, & Pl. Also find the three quantities: Qh, Qm, & Ql.
- Find CS for each group or in each market.
- Give your own conclusion of whether PD was “good” or “bad” to each group. In your opinion as an economist, was PD “good” or “bad” in this specific scenario?
Solutions
Expert Solution
(a). The monopolist do not price discriminate between three markets. We find equilibirium prices, output and consumer surplus in following ways:
- We shall aggregate the demand function of all three groups of
customer. Consider:
- Consumer 1 = Ph = 24-2Qh ----> Qh = 1/2(24-Ph).
- Consumer 2 = Ph = 20-2Qm -----> Qm = 1/2(20-Pm)
- Consumer 3 = Pl = 16-2Ql ------> Ql = 1/2(16-Pl)
- Summation of above (We shall remove the market segmentation by denoting them all by P and Q only)


- Inversing the market demand, we get:

- Now, the profit maximization requires first order conditions. Consider:

- First order condition requires

- We know MC is constant and equivalent to MC = $14.
The MR is 

- Equating MR and MC, we find equilibirium output


- Then, equilibirium or uniform price charged by Monopolist is:


The monopolist charges price $17 and sells 1.5 units in the big market.
Now, we shall calculate quantity sold at uniform price in all three market and respective consumer surplus.
- High income Group market.
- The market demand is given by : Ph = 24-2Qh.
- Using uniform prices, we get: 17 = 24-2Qh --------> Qh = 3.5 units.
- Consumer Surplus =

- In this case, maximum price is 24 when quantity sold is zero.(U can apply graph Logic)
- CS = 1/2( 24-17)*(3.5) -----> 12.25.
- Thus, consumer surplus is 12.25.
- Middle income group market.
- The market demand is : Pm = 20-2Qm
- The quantity sold is : 17=20-2Qm -----> 1.5 units.
- Maximum price which monopolist can charge is at zero output sold i.e. P = 20.
- So, consumer surplus would be : 0.5(20-17)*1.5 ------->2.25
- The consumer surplus is $2.25.
- Low income group market.
- The market demand curve is : Pl = 16-2Ql.
- The quantity sold at uniform prices would be : 17 = 16-2Ql------>(-0.5)
- The negative means no quantity would be sold.
- The consumer surplus would be in negative i.e 0.5(16-17)*0.5 = 0.25.
(b) Now, Monopolist price discriminate between all market. He will equate the MR and MC of each market separately to get equilibirium price and quantity of each market. Consider,
- High income group.
- TR = P.Q = (24-2Qh)Qh ----> 24Qh - 2Qh2.
- Then, MR = 24 - 4Qh
- Equating MR with the MC for profit maximizing price and quantity. (MC =14)
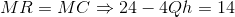
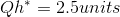
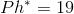
- Consumer surplus would be:
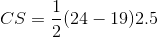

- Middle income group.
- Low income group:
(c) To check whether price discrimination was good or not, we need to analyse aggregate profit and sales pre and post price discrimination. We shall be doing this by aggregating all markets. Consider,
- High Income Group.
- Before price discrimination, Price were $ 17 and Quantity sold was 3.5 unit.
- Post price discrimination, prices increased by $2 and unit sold decreased by 1 unit. Also, consumer surplus has declined. From economic point of view, this may not be good as the welfare is reduced due to increase in deadweight loss and fall in consumer plus.
- Middle income group.
- This group has neither benefitted nor lossed from pre and post price discrimination. Since, price and unit sold are same and consumer surplus as well.
- Low income group.
- This group has benefitted from lower prices due to price discrimination. The prices reduced by$2 post discrimination and unit sold increased to 0.5 (None in pre-discrimination period)
- Overall, Pre-Price discrimination.
- Aggregate sales of all three markets = 3.5+1.5+(-0.5) = 4.5 units.
- Aggregate profit is : (P-MC)Q-----> (17-14)4.5 = $13.5
- Overall, Post price discrimination.
- Aggregate sales of all three markets = 2.5+1.5+0.5 = 4.5 units.
- Aggregate profit for all is: (19-14)2.5 + (17-14)1.5 + (15-14)0.5 = $17.5
- We can clearly see that though monopoly sold same quantity post and pre price discrimination, her total profits were more in the price discrimination by $4. This means Price-discrimination is profitable.
- However, from welfare point of view, the PD is not good as it has reduced aggregate consumer surplus from Pre-price discrimination period to Post price discrimination period.
Related Solutions
A monopolist faces three groups of customers (or three markets) such that: Demand of the high-income...
A monopolist faces 300 customers divided into 3 different groups: 1. High-Demand customers each have a...
. A monopolist faces two types of consumers: low demand consumers and high demand consumers. A...
Suppose a monopolist faces two groups of consumers. Group 1 has a demand given by P1...
Suppose a software monopolist faces two markets for its software, students and professionals. The demand curve...
Suppose a monopolist faces two markets with the following demand curves: Market 1: ?1 (?1 )...
4. Suppose a monopolist faces two markets with the following demand curves: Market 1: ?1(?1) =...
A monopolist faces two totally separated markets with inverse demand p=200−2?1 and p=320−4?2 respectively. The monopolist...
A monopolist faces the inverse demand for its output: p = 30 − Q. The monopolist...
a) A monopolist faces two totally separated markets with inverse demand p=100 – qA and p=160−2qB...
- Assignment # 6: Chain of Custody Roles and Requirements Learning Objectives and Outcomes Describe the requirements...
- What biological, psychological, and social cultural influences affect our experience of pain? How do placebos, distraction,...
- A spherical object has an outside diameter of 60.0cm . Its outer shell is composed of...
- Write the code for binary min heap in c++ .
- Starting with a 30% (w/w) solution of hydrogen peroxide, how many mL would you need to...
- An object with a density of 761.0 kg/m3 and a mass of 1399.0 kg is thrown...
- British Columbia Lumber has a raw lumber division and a finished lumber division. The variable costs...

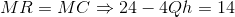
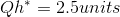

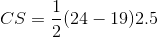











 Rahul Sunny answered 1 year ago
Rahul Sunny answered 1 year ago